Asymptotic Distribution of Quasi-Normal Modes for Kerr de Sitter Black Holes
|
|
|
- Holger Arnfinn Rød
- 4 år siden
- Visninger:
Transkript
1 Ann. Henri Poincaré 13 (2012), c 2012 Springer Basel AG /12/ published online January 25, 2012 DOI /s y Annales Henri Poincaré Asymptotic Distribution of Quasi-Normal Modes for Kerr de Sitter Black Holes Semyon Dyatlov Abstract. We establish a Bohr Sommerfeld type condition for quasinormal modes of a slowly rotating Kerr de Sitter black hole, providing their full asymptotic description in any strip of fixed width. In particular, we observe a Zeeman-like splitting of the high multiplicity modes at a = 0 (Schwarzschild de Sitter), once spherical symmetry is broken. The numerical results presented in Appendix B show that the asymptotics are in fact accurate at very low energies and agree with the numerical results established by other methods in the physics literature. We also prove that solutions of the wave equation can be asymptotically expanded in terms of quasi-normal modes; this confirms the validity of the interpretation of their real parts as frequencies of oscillations, and imaginary parts as decay rates of gravitational waves. Quasi-normal modes (QNMs) of black holes are a topic of continued interest in theoretical physics: from the assical interpretation as ringdown of gravitational waves [11] to the recent investigations in the context of string theory [33]. The ringdown 1 plays a role in experimental projects aimed at the detection of gravitational waves, such as LIGO [1]. See [35] for an overview of the vast physics literature on the topic and [6,36,37,53] for some more recent developments. In this paper we consider the Kerr de Sitter model of a rotating black hole and assume that the speed of rotation a is small; for a = 0, one gets the stationary Schwarzschild de Sitter black hole. The de Sitter model corresponds to assuming that the cosmological constant Λ is positive, which is consistent with the current Lambda-CDM standard model of cosmology. 1 Here is an irresistible quote of Chandrasekhar... we may expect that any initial perturbation will, during its last stages, decay in a manner characteristic of the black hole itself and independent of the cause. In other words, we may expect that during these last stages, the black hole emits gravitational waves with frequencies and rates of damping that are characteristic of the black hole itself, in the manner of a bell sounding its last dying notes.
2 1102 S. Dyatlov Ann. Henri Poincaré A rigorous definition of quasi-normal modes for Kerr de Sitter black holes was given using the scattering resolvent in [26]. In Theorem 1 below we give an asymptotic description of QNMs in a band of any fixed width, that is, for any bounded decay rate. The result confirms the heuristic analogy with the Zeeman effect: the high multiplicity modes for the Schwarzschild black hole split. Theorem 2 confirms the standard interpretation of QNMs as complex frequencies of exponentially decaying gravitational waves; namely, we show that the solutions of the scalar linear wave equation in the Kerr de Sitter background can be expanded in terms of QNMs. In the mathematics literature, quasi-normal modes of black holes were studied by Bachelot, Motet-Bachelot, and Pravica [3 5, 39] using the methods of scattering theory. QNMs of Schwarzschild de Sitter metric were then investigated by Sá Barreto Zworski [41], resulting in the lattice of pseudopoles given by (0.3) below. For this case, Bony Häfner [10] established polynomial cutoff resolvent estimates and a resonance expansion, Melrose Sá Barreto Vasy [38] obtained exponential decay for solutions to the wave equation up to the event horizons, and Dafermos Rodnianski [17] used physical space methods to obtain decay of linear waves better than any power of t. Quasi-normal modes for Kerr de Sitter were rigorously defined in [26] and exponential decay beyond event horizons was proved in [27]. Vasy [50] has recently obtained a microlocal description of the scattering resolvent and in particular recovered the results of [26, 27] on meromorphy of the resolvent and exponential decay; see [50, Appendix] for how his work relates to [26]. The crucial component for obtaining exponential decay was the work of Wunsch Zworski [52] on resolvent estimates for normally hyperbolic trapping. We add that there have been many papers on decay of linear waves for Schwarzschild and Kerr black holes see [2, 9, 18, 19, 22, 23, 28, 29, 46, 47, 49] and references given there. In that case the cosmological constant is 0 (unlike in the de Sitter case, where it is positive), and the methods of scattering theory are harder to apply because of an asymptotically Euidean infinity. Theorem 1. Fix the mass M 0 of the black hole and the cosmological constant Λ. (SeeSect.1.1 for details.) Then, there exists a constant a 0 > 0 such that for a <a 0 and each ν 0, there exist constants C ω,c m 2 such that the set of quasi-normal modes ω satisfying Re ω>c ω, Im ω> ν 0 (0.1) coincides modulo O( ω ) with the set of pseudopoles ω = F(m, l, k), m,l,k Z, 0 m C m, k l. (0.2) (Since the set of QNMs is symmetric with respect to the imaginary axis, one also gets an asymptotic description for Reω negative. Also, by [26, Theorem 4], 2 As in [26], the indices ω,m,... next to constants, symbols, operators, and functions do not imply differentiation.
3 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter 1103 all QNMs lie in the lower half-plane). Here F is a complex valued assical symbol 3 of order 1 in the (l, k) variables, defined and smooth in the cone {m [0,C m ], k l} R 3. The principal symbol F 0 of F is real-valued and independent of m; moreover, 1 9ΛM 2 F = 0 3 [(l +1/2) i(m +1/2)] + O(l 1 ) for a =0, (0.3) 3M 0 ( k F 0 )(m, ±k, k) = (2 + 9ΛM 0 2 )a 27M0 2 + O(a 2 ). (0.4) The pseudopoles (0.2) can be computed numerically; we have implemented this computation in a special case l k = O(1) and compared the pseudopoles with the QNMs computed by the authors of [6]. The results are described in Appendix B. One should note that the quantization condition of [41] was stated up to O(l 1 ) error, while Theorem 1 has error O(l ); we demonstrate numerically that increasing the order of the quantization condition leads to a substantially better approximation. Another difference between (0.2) and the quantization condition of [41] is the extra parameter k, resulting from the lack of spherical symmetry of the problem. In fact, for a = 0 each pole in (0.3) has multiplicity 2l +1; for a 0 this pole splits into 2l + 1 distinct QNMs, each corresponding to its own value of k, the angular momentum with respect to the axis of rotation. (The resulting QNMs do not coincide for small values of a, as illustrated by (0.4)). In the physics literature this is considered an analog of the Zeeman effect. Since the proof of Theorem 1 only uses microlocal analysis away from the event horizons, it implies estimates on the cutoff resolvent polynomial in ω (Proposition 1.3). Combining these with the detailed analysis away from the trapped set (and in particular near the event horizons) by Vasy [50], we obtain estimates on the resolvent on the whole space (Proposition 1.2). These in turn allow a contour deformation argument leading to an expansion of waves in terms of quasinormal modes. Such expansions have a long tradition in scattering theory going back to Lax Phillips and Vainberg see [45] for the strongly trapping case and for references. For Schwarzschild de Sitter black holes, a full expansion involving infinite sums over quasinormal modes was obtained in [10] (seealso[13] for simpler expansions involving infinite sums over resonances). The next theorem presents an expansion of waves for Kerr de Sitter black holes in the same style as the Bony Häfner expansion: Theorem 2. Under the assumptions of Theorem 1, take ν 0 > 0 such that for some ε>0, every QNM ω has Im ω + ν 0 >ε. (Such ν 0 exists and can be chosen arbitrarily large, as the imaginary parts of QNMs lie within O( a +l 1 ) of those in (0.3)). Then, for s large enough depending on ν 0, there exists a constant C such that every solution u to the Cauchy problem on the Kerr de Sitter 3 Here symbol means a microlocal symbol as in for example [48, Section 8.1]. For the proofs, however, we will mostly use semiassical symbols, as defined in Sect. 2.1.
4 1104 S. Dyatlov Ann. Henri Poincaré space g u =0, u t =0 = f 0 H s (X δ ), t u t =0 = f 1 H s 1 (X δ ), (0.5) where X δ =(r δ, r + + δ) S 2 is the space slice, t is the time variable, and δ>0 is a small constant (see Sect. 1.1 for details), satisfies for t > 0, u(t ) Π ν0 (f 0,f 1 )(t ) H1 (X δ ) Ce ν0t ( f 0 H s + f 1 H s 1). (0.6) Here Π ν0 (f 0,f 1 )(t )= e it ω (t ) j Π ω,j (f 0,f 1 ); (0.7) Im ω> ν 0 0 j<j ω the outer sum is over QNMs ω, J ω is the algebraic multiplicity of ω as a pole of the scattering resolvent, and Π ω,j are finite rank operators mapping H s (X δ ) H s 1 (X δ ) C (X δ ). Moreover, for ω large enough (that is, for all but a finite number of QNMs in the considered strip), J ω =1, Π ω,0 has rank one, and Π ω,0 Hs (X δ ) H s 1 (X δ ) H 1 (X δ ) C ω N s. Here N is a constant depending on ν 0,butnotons; therefore, the series (0.7) converges in H 1 for s>n+2. The proofs start with the Teukolsky separation of variables used in [26], which reduces our problem to obtaining quantization conditions and resolvent estimates for certain radial and angular operators (Propositions 1.5 and 1.6). These conditions are stated and used to obtain Theorems 1 and 2 in Sect. 1. Also, at the end of Sect. 1.2 we present the separation argument in the simpler special case a = 0, for convenience of the reader. In the spherically symmetric case a = 0, the angular problem is the eigenvalue problem for the Laplace Beltrami operator on the round sphere. For a 0, the angular operator P θ is not self-adjoint; however, in the semiassical scaling it is an operator of real principal type with completely integrable Hamiltonian flow. We can then use some of the methods of [31] to obtain a microlocal normal form for h 2 P θ ; since our perturbation is O(h), we are able to avoid using analyticity of the coefficients of P θ. The quantization condition we get is global, similarly to [51]. The proof is contained in Sect. 3; ituses various tools from semiassical analysis described in Sect. 2. To complete the proof of the angular quantization condition, we need to extract information about the joint spectrum of h 2 P θ and hd ϕ from the microlocal normal form; for that, we formulate a Grushin problem for several commuting operators. The problem that needs to be overcome here is that existence of joint spectrum is only guaranteed by exact commutation of the original operators, while semiassical methods always give O(h ) errors. This complication does not appear in [31,32] as they study the spectrum of a single operator, nor in earlier works [12, 51] on joint spectrum of differential operators, as they use spectral theory of self-adjoint operators. Since this part of the construction can be formulated independently of the rest, we describe Grushin problems for several operators in an abstract setting in Appendix A.
5 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter 1105 The radial problem is equivalent to one-dimensional semiassical potential scattering. The principal part of the potential is real-valued and has a unique quadratic maximum; the proof of the quantization condition follows the methods developed in [16,40,42]. In [16], the microlocal behavior of the principal symbol near a hyperbolic critical point is studied in detail; however, only self-adjoint operators are considered and the phenomenon that gives rise to resonances in our case does not appear. The latter phenomenon is studied in [40] and [42]; our radial quantization condition, proved in Sect. 4, can be viewed as a consequence of [40, Theorems 2 and 4]. However, we do not compute the scattering matrix, which simplifies the calculations; we also avoid using analyticity of the potential near its maximum and formulate the quantization condition by means of real microlocal analysis instead of the action integral in the complex plane. As in [40], we use analyticity of the potential near infinity and the exact WKB method to relate the microlocal approximate solutions to the outgoing condition at infinity; however, the construction is somewhat simplified compared to [40, Sections 2 and 3] using the special form of the potential. It would be interesting to see whether our statements still hold if one perturbs the metric, or if one drops the assumption of smallness of a. Near the event horizons, we rely on [26, Section 6], which uses a perturbation argument (thus smallness of a) and analyticity of the metric near the event horizons. Same applies to Sect. 4.2 of the present paper; the exact WKB construction there requires analyticity and Proposition 4.2 uses that the values ω ± defined in (4.9) are nonzero, which might not be true for large a. However, it is very possible that the construction of the scattering resolvent of [50] can be used instead. The methods of [50] are stable under rather general perturbations, see [50, Section 2.7], and apply in particular to Kerr de Sitter black holes with a satisfying [50, (6.12)]. A more serious problem is the fact that Theorem 1 is a quantization condition, and thus is expected to hold only when the geodesic flow is completely integrable, at least on the trapped set. For large a, the separation of variables of Sect. 1.2 is still valid, and it is conceivable that the global structure of the angular integrable system in Sect. 3.2 and of the radial barrier-top Schrödinger operator in Sect. 4.1 would be preserved, yielding Theorem 1 in this case. Even then, the proof of Theorem 2 no longer applies as it relies on having gaps between the imaginary parts of resonances, which might disappear for large a. However, a generic smooth perturbation of the metric supported near the trapped set will destroy complete integrability and thus any hope of obtaining Theorem 1. One way of dealing with this is to impose the condition that the geodesic flow is completely integrable on the trapped set. In principle, the global analysis of [51] together with the methods for handling O(h) nonself-adjoint perturbations developed in Sect. 3 and Appendix A should provide the quantization condition in the direction of the trapped set, while the barrier-top resonance analysis of Sect. 4.3 should handle the transversal directions. However, without separation of variables one might need to merge these
6 1106 S. Dyatlov Ann. Henri Poincaré methods and construct a normal form at the trapped set which is not presented here. Another possibility is to try to establish Theorem 2 without a quantization condition, perhaps under the (stable under perturbations) assumption that the trapped set is normally hyperbolic as in [52]. However, this will require to rethink the contour deformation argument, as it is not ear which contour to deform to when there is no stratification of resonances by depth, corresponding to the parameter m in Theorem Proofs of Theorems 1 and Kerr de Sitter Metric First of all, we define Kerr de Sitter metric and briefly review how solutions of the wave equation are related to the scattering resolvent; see also [26, Section 1] and [50, Section 6]. The metric is given by g = ρ 2( dr 2 ) + dθ2 Δ θ sin 2 θ Δ r Δ θ (1 + α) 2 ρ 2 (adt (r2 + a 2 ) dϕ) 2 Δ r + (1 + α) 2 ρ 2 (dt a sin2 θdϕ) 2. Here θ [0,π]andϕ R/2πZ are the spherical coordinates on S 2 and r, t take values in R; M 0 is the mass of the black hole, Λ is the cosmological constant, and a is the angular momentum; ) Δ r =(r 2 + a 2 ) (1 Λr2 2M 0 r, Δ θ =1+αcos 2 θ, 3 ρ 2 = r 2 + a 2 cos 2 θ, α = Λa2 3. The metric in the (t, r, θ, ϕ) coordinates is defined for Δ r > 0; we assume that this happens on an open interval r (r,r + ), where r ± are two of the roots of the fourth order polynomial equation Δ r (r) = 0. The metric becomes singular at r = r ± ; however, this apparent singularity goes away if we consider the following version of the Kerr-star coordinates (see [18, Section 5.1] and [47]): t = t F t (r), ϕ = ϕ F ϕ (r), (1.1) with the functions F t,f ϕ blowing up like c ± log r r ± as r approaches r ±. One can choose F t,f ϕ so that the metric continues smoothly across the surfaces {r = r ± }, called event horizons, to M δ = R t X δ,x δ =(r δ, r + + δ) S 2, with δ>0 is a small constant. Moreover, the surfaces {t =const} are spacelike, while the surfaces {r =const} are timelike for r (r,r + ), spacelike for r [r,r + ], and null for r {r,r + }. See [26, Section 1], [27, Section 1.1], or [50, Section 6.4] for more information on how to construct F t,f ϕ with these properties.
7 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter 1107 Let g be the d Alembert Beltrami operator of the Kerr de Sitter metric. Take f H s 1 (M δ ) for some s 1, and furthermore assume that f is supported in {0 t 1}. Then, since the boundary of M δ is spacelike and every positive time oriented vector at M δ points outside of M δ, by the theory of hyperbolic equations (see for example [18, Proposition 3.1.1] or [48, Sections 2.8 and 7.7]) there exists unique solution u H s loc (M δ) to the problem g u = f, supp u {t 0}. (1.2) We will henceforth consider the problem (1.2); the Cauchy problem (0.5)canbe reduced to (1.2) as follows. Assume that u solves (0.5) with some f 0 H s (X δ ), f 1 H s 1 (X δ ). Take a function χ C (R) such that supp χ {t > 0} and supp(1 χ) {t < 1}; then, χ(t )u solves (1.2) with f =[ g,χ]u supported in {0 t 1} and the H s 1 norm of f is controlled by f 0 H s + f 1 H s 1. Since the metric is stationary, there exists a constant C e such that every solution u to (1.2) grows slower than e (Ce 1)t ; see [26, Proposition 1.1]. Therefore, the Fourier Laplace transform û(ω) = e iωt u(t )dt is well defined and holomorphic in {Im ω C e }. Here both u(t )andu(ω) are functions on X δ. Moreover, if ˆf(ω) is the Fourier Laplace transform of f, then P g (ω)û(ω) =ρ 2 ˆf(ω), Im ω Ce, (1.3) where P g (ω) is the stationary d Alembert Beltrami operator, obtained by replacing D t with ω in ρ 2 g. (The ρ 2 factor will prove useful in the next subsection). Finally, since f is supported in {0 t 1}, the function ˆf(ω) is holomorphic in the entire C, and ω s 1 ˆf(ω) H s 1 ω 1 (X δ) C f H s 1 (M δ ) (1.4) for Im ω bounded by a fixed constant. Here H s 1 h, h>0, is the semiassical Sobolev space, consisting of the same functions as H s 1, but with norm hd s 1 f L 2 instead of D s 1 f L 2. If P g (ω) was, say, an elliptic operator, then the Eq. (1.3) would have many solutions; however, because of the degeneracies occurring at the event horizons, the requirement that û H s acts as a boundary condition. This situation was examined in detail in [50]; the following proposition follows from [50, Theorem 1.2 and Lemma 3.1] (see [26, Proposition 1.2] for the cutoff version): Proposition 1.1. Fix ν 0 > 0. Then,fors large enough depending on ν 0, there exists a family of operators (called the scattering resolvent) R(ω) :H s 1 (X δ ) H s (X δ ), Im ω ν 0, meromorphic with poles of finite rank and such that for u solving (1.2), we have û(ω) =R(ω) ˆf(ω), Im ω C e. (1.5)
8 1108 S. Dyatlov Ann. Henri Poincaré Note that even though we originally defined the left-hand side of (1.5) for Im ω C e, the right-hand side of this equation makes sense in a wider region Im ω ν 0, and in fact in the entire complex plane if f is smooth. The idea now is to use Fourier inversion formula u(t )= 1 2π Im ω=c e e iωt R(ω) ˆf(ω)dω (1.6) and deform the contour of integration to {Im ω = ν 0 } to get exponential decay via the e iωt factor. We pick up residues from the poles of R(ω) when deforming the contour; therefore, one defines quasi-normal modes as the poles of R(ω). Our ability to deform the contour and estimate the resulting integral depends on having polynomial resolvent estimates. To formulate these, let us give the technical. Definition 1.1. Let h>0 be a parameter and R(ω; h):h 1 H 2, ω U(h) C, be a meromorphic family of operators, with H j Hilbert spaces. Let also Ω(h) U(h) beopenandz(h) C be a finite subset; we allow elements of Z(h) to have multiplicities. We say that the poles of R in Ω(h) are simple with a polynomial resolvent estimate and given modulo O(h )byz(h), if for h small enough, there exist maps Q and Π from Z(h) toc and the algebra of bounded operators H 1 H 2, respectively, such that: for each ω Z(h), ω = Q( ω ) is a pole of R, ω ω = O(h ), and Π( ω ) is a rank one operator; there exists a constant N such that Π( ω ) H1 H 2 = O(h N )foreach ω Z(h) and, moreover, R(ω; h) = ω Z(h) Π( ω ) ω Q( ω ) + O H 1 H 2 (h N ), ω Ω(h). In particular, every pole of R in Ω(h) lies in the image of Q. The quantization condition and resolvent estimate that we need to prove Theorems 1 and 2 are contained in Proposition 1.2. Fix ν 0 > 0 and let h>0 be a parameter. Then, for a small enough (independently of ν 0 ), the poles of R(ω) in the region Im ω <ν 0, h 1 < Re ω < 2h 1, (1.7) are simple with a polynomial resolvent estimate and given modulo O(h ) by ω = h 1 F ω (m, hl, hk; h), m,l,k Z, 0 m C m, C 1 (1.8) l hl C l, k l. Here C m and C l are some constants and F ω (m, l, k; h) is a assical symbol: F ω (m, l, k; h) h j Fj ω (m, l, k). j 0
9 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter 1109 The principal symbol F ω 0 is real-valued and independent of m; moreover, F ω (m, l, k; 1 9ΛM 2 h) = 0 3 ( l + h/2 ih(m +1/2)) + O(h 2 ) for a =0, 3M 0 ( kf 0 ω )(m, ± k, k) = (2 + 9ΛM 0 2 )a 27M0 2 + O(a 2 ). Finally, if we consider R(ω) as a family of operators between the semiassical Sobolev spaces H s 1 h Hh s, then the constant N in Definition 1.1 is independent of s. Theorem 1 follows from here almost immediately. Indeed, since R(ω) is independent of h, eachf ω j is homogeneous in ( l, k) variables of degree 1 j; we can then extend this function homogeneously to the cone k l and define the (nonsemiassical) symbol F(m, l, k) j 0 F ω j (m, l, k). Note that F(m, l, k) =h 1 F ω (m, hl, hk; h)+o(h ) whenever C 1 l hl C l. We can then cover the region (0.1) for large C ω with the regions (1.7) fora sequence of small values of h to see that QNMs in (0.1) are given by (0.2) modulo O( ω ). Now, we prove Theorem 2. Letu be a solution to (1.2), with f H s 1 and s large enough. We aim that one can deform the contour in (1.6) toget u(t )=i Res ω= ω [e iωt R(ω) ˆf(ω)] + 1 e iωt R(ω) 2π ˆf(ω)dω. Im ω> ν 0 Im ω= ν 0 (1.9) The series in (1.9)isoverQNMs ω; all but a finite number of them in the region {Im ω> ν 0 } are equal to Q( ω ) for some ω given by (0.2) and the residue in this case is e i ωt Π( ω ) ˆf( ω). Here Q and Π are taken from Definition 1.1. Now, by (1.4), we have Π( ω ) ˆf( ω) H 1 C ω N s f H s 1; R(ω) ˆf(ω) H 1 C ω N s f H s 1, Im ω = ν 0, for some constant N independent of s; therefore, for s large enough, the series in (1.9) converges in H 1 and the H 1 norm of the integral in (1.9) canbe estimated by Ce ν0t f H s 1, thus proving Theorem 2. To prove (1.9), take small h > 0. There are O(h 2 ) QNMs in the region (1.7); therefore, by pigeonhole principle we can find ω 0 (h) [h 1, 2h 1 ] such that there are no QNMs h 2 -ose to the segments γ ± (h) ={Re ω = ±ω 0 (h), ν 0 Im ω C e }. Then, R(ω) ˆf(ω) H 1 = O(h s N 4 )onγ ± (h); we can now apply the residue theorem to the rectangle formed from γ ± (h) and segments of the lines {Im ω = C e }, {Im ω = ν 0 }, and then let h 0.
10 1110 S. Dyatlov Ann. Henri Poincaré 1.2. Separation of Variables First of all, using [50, (A.2), (A.3)], we reduce Proposition 1.2 to the following 4 Proposition 1.3. Take δ>0 and put K δ =(r + δ, r + δ) S 2, R g (ω) =1 Kδ R(ω)1 Kδ : L 2 (K δ ) H 2 (K δ ). Then, for a small enough 5 and fixed ν 0, the poles of R g (ω) in the region (1.7) are simple with a polynomial resolvent estimate L 2 L 2 and given modulo O(h ) by (1.8). Furthermore, by [50, Proposition A.1] the family of operators R g (ω) coincides with the one constructed in [26, Theorem 2], if the functions F t,f ϕ in (1.1) are chosen so that (t, ϕ) =(t,ϕ )inr t K δ. We now review how the construction of R g (ω) in[26] works and reduce Proposition 1.3 to two separate spectral problems in the radial and the angular variables. For the convenience of reader, we inude the simpler separation of variables procedure for the case a = 0 at the end of this section. First of all, the operator P g (ω) is invariant under the rotation ϕ ϕ + s; therefore, the spaces D k = Ker(D ϕ k) of functions of angular momentum k Z are invariant under both P g (ω) andr g (ω). In [26], we construct R g (ω) by piecing together the restrictions R g (ω, k) =R g (ω) D k for all k. Then, Proposition 1.3 follows from Proposition 1.4. Under the assumptions of Proposition 1.3, there exists a constant C k such that for each k Z, 1. if h k >C k, then R g (ω, k) has no poles in the region (1.7) and its L 2 L 2 norm is O( k 2 ); (This is a reformulation of [26, Proposition 3.3]). 2. if h k C k, then the poles of R g (ω, k) in the region (1.7) are simple with a polynomial resolvent estimate L 2 L 2 and given modulo O(h ) by (1.8), with this particular value of k. Now, we recall from [26, Section 1] that the restriction of P g (ω) tod k has the form 6 P r (ω, k)+p θ (ω) D k, where (1 + α)2 P r (ω, k) =D r (Δ r D r ) ((r 2 + a 2 )ω ak) 2, (1.10) Δ r P θ (ω) = 1 sin θ D (1 + α)2 θ(δ θ sin θd θ )+ Δ θ sin 2 θ (aω sin2 θ D ϕ ) 2 (1.11) are differential operators in r and (θ, ϕ), respectively. Then, R g (ω, k) isconstructed in [26, Proof of Theorem 1] using a certain contour integral [26, (2.1)] 4 One could also try to apply the results of [20] here, but we use the slightly simpler construction of [50, Appendix], exploiting the fact that we have information on the exact cutoff resolvent. 5 The smallness of a is implied in all following statements. 6 The operator P g(ω) of [26] differs from our operator by the conjugation done in [50, Appendix]; however, the two coincide in K δ.
11 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter 1111 and the radial and angular resolvents R r (ω, λ, k) :L 2 comp(r,r + ) Hloc(r 2,r + ), R θ (ω, λ) :L 2 (S 2 ) H 2 (S 2 ),λ C; R r is a certain right inverse to P r (ω, k)+λ, while R θ is the inverse to P θ (ω) λ; we write R θ (ω, λ, k) =R θ (ω, λ) D k. Recall that both R r and R θ are meromorphic families of operators, as defined in [26, Definition 2.1]; in particular, for a fixed value of ω, these families are meromorphic in λ with poles of finite rank. By definition of R g (ω, k), a number ω C is a pole of this operator if and only if there exists λ C such that (ω, λ, k) is a pole of both R r and R θ. Now, for small h>0 we put ω = h Re ω, ν =Imω, λ = h 2 Re λ, μ = h Im λ, k = hk; (1.12) the assumptions of Proposition 1.4(2) imply that 1 ω 2, ν ν 0,and k C k. Moreover, [26, Proposition 3.4] suggests that under these assumptions, all values of λ for which (ω, λ, k) is a pole of both R r and R θ have to satisfy λ, μ C λ, for some constant C λ. We are now ready to state the quantization conditions and resolvent estimates for R r and R θ ; the former is proved in Sect. 4 and the latter, in Sect. 3. Proposition 1.5 (Radial lemma). Let C λ be a fixed constant and put K r = (r + δ, r + δ). Then, the poles of 1 Kr R r (ω, λ, k)1 Kr as a function of λ in the region 1 < ω <2, ν <ν 0, k <C k, λ, μ <C λ, (1.13) are simple with polynomial resolvent estimate L 2 L 2 (in the sense of Definition 1.1) and given modulo O(h ) by λ + ih μ = F r (m, ω, ν, k; h), m Z, 0 m C m, (1.14) for some constant C m. The principal part F0 r of the assical symbol F r is real-valued, independent of m and ν, and F r = [ ih(m +1/2) + 3 3M 0 1 9ΛM 2 0 ( ω + ih ν)] 2 + O(h 2 ) for a =0, F0 r ( ω, k) = 27M ΛM0 2 ω 2 6a k ω 1 9ΛM0 2 + O(a 2 ). In particular, for ω, k satisfying (1.13), every pole λ satisfies λ >εfor some constant ε>0. Proposition 1.6 (Angular lemma). Let C θ be a fixed constant. Then, the poles of R θ (ω, λ, k) as a function of λ in the region 1 < ω <2, ν <ν 0, k <C k, C 1 θ < λ <C θ, μ <C θ, (1.15) are simple with polynomial resolvent estimate L 2 L 2 and given modulo O(h ) by λ + ih μ = F θ (hl, ω, ν, k; h), l Z, max( k,c 1 l ) hl C l, (1.16)
12 1112 S. Dyatlov Ann. Henri Poincaré Figure 1. The contour γ and interaction between radial poles (denoted by dots) and angular poles (denoted by asterisks) for some constant C l. The principal part F0 θ of the assical symbol F θ is real-valued, independent of ν, and F θ = l( l + h)+o(h ) for a =0. Moreover, F0 θ (± k, ω, k) =(1+α) 2 ( k a ω) 2, lf 0 θ (± k, ω, k) =±2 k + O(a 2 ), and consequently, kf 0 θ (± k, ω, k) = 2a ω + O(a 2 ). Combining Propositions 1.5 and 1.6 with the results of [26], we get Proof of Proposition 1.4. We let F ω (m, l, k; h) be the solution ω + ih ν to the equation F r (m, ω, ν, k; h) =F θ ( l, ω, ν, k; h). (1.17) We can see that this equation has unique solution by writing F r F θ = F +ihf and examining the principal parts of the real-valued symbols F, F for a =0. The idea now is to construct an admissible contour in the sense of [26, Definition 2.3]; e.g., a contour that separates the sets of poles (in the variable λ) ofr r and R θ from each other; then, [26, (2.1)] provides a formula for R g (ω, k), which can be used to get a resolvent estimate. We will use the method of proof of [26, Proposition 3.4]. Take the contour γ introduced there (Fig. 1), for l 2 = C λ h 1, l 1 = L = C λ h 2,andC λ some
13 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter 1113 large constant. Then, we know that all angular poles are to the right of γ (in Γ 2 ). Moreover, the only radial poles to the right of γ lie in the domain { Im λ l 2, λ L} and they are contained in the set {λ r 0,...,λ r C m } for some constant C m, where λ r m(ω, k) is the radial pole corresponding to h 2 F r (m, ω, ν, k; h). In particular, those radial poles are contained in U λ = {C 1 θ < λ <C θ, μ C θ }, for some constant C θ. Assume that ω is not a pole of R g (ω, k); then, we can consider the admissible contour composed of γ and the cires γ m,0 m C m, enosing λ r m(ω, k), but none of the other poles of R r or R θ. Using the meromorphic decomposition of R r at λ r m and letting its principal part be Π r m/(λ λ r m), we get R g (ω, k) = Π r m(ω, k) R θ (ω, λ r m(ω, k),k) m + 1 R r (ω, λ, k) R θ (ω, λ, k) dλ. (1.18) 2πi γ Here we only inude the poles λ r m lying to the right of γ; one might need to change l 1 in the definition of γ a little bit in case some λ r m comes ose to γ. The integral in (1.18) is holomorphic and bounded polynomially in h, bythe bounds for R r given by Proposition 1.5, together with the estimates in the proof of [26, Proposition 3.4]. Now, the poles of R θ in U λ are given by (1.16); let λ θ l (ω, k) be the pole corresponding to h 2 F θ (hl, ω, ν, k; h) and Π θ l /(λ λθ l ) be the principal part of the corresponding meromorphic decomposition. Then, the resolvent estimates on R θ given by Proposition 1.6 together with (1.18) imply R g (ω, k) =Hol(ω)+ m,l Π r m(ω, k) Π θ l (ω, k) λ r m(ω, k) λ θ. (1.19) l (ω, k) Here Hol(ω) is a family of operators holomorphic in ω and bounded polynomially in h. Moreover, λ r m(ω, k) λ θ l (ω, k) =h 2 (F r (m, ω, ν, k; h) F θ (hl, ω, ν, k; h)) + O(h ); (1.20) therefore, the equation λ r m(ω, k) λ θ l (ω, k) = 0 is an O(h ) perturbation of (1.17) and it has a unique solution ω m,l (k), which is O(h ) ose to h 1 F ω (m, hl, k; h). Finally, in the region (1.7) we can write by (1.19) R g (ω, k) =Hol(ω)+ m,l Π m,l (k) ω ω m,l (k), with Hol(ω) asaboveandπ m,l (k) being the product of a coefficient polynomially bounded in h with (Π r m Π θ l )(ω m,l(k),k); this finishes the proof.
14 1114 S. Dyatlov Ann. Henri Poincaré Finally, let us present the simplified separation of variables for the case a = 0, namely the Schwarzschild de Sitter metric: g = Δ r r 2 dt2 r2 dr 2 r 2 (dθ 2 +sin 2 θdϕ 2 ), Δ r Δ r = r 2( 1 Λr2 3 ) 2M 0 r. Note that dθ 2 +sin 2 θdϕ 2 is just the round metric on the unit sphere. The metric decouples without the need to take Fourier series in ϕ; the stationary d Alembert Beltrami operator has the form P g (ω) =P r (ω)+p θ, where P r (ω) =D r (Δ r D r ) r4 ω 2, Δ r P θ = 1 sin θ D θ(sin θd θ )+ D2 ϕ sin 2 θ. Here P θ is the Laplace Beltrami operator on the round sphere; it is self-adjoint (thus, no need for the contour integral construction of [26, Section 2]) and is known to have eigenvalues l(l + 1), where l 0. Each such eigenvalue has multiplicity 2l + 1, corresponding to the values l,...,l of the ϕ-angular momentum k. The angular Lemma 1.6 follows immediately. (We nevertheless give a more microlocal explanation in this case at the end of Sect. 3.1.) One can now decompose L 2 into an orthogonal sum of the eigenspaces of P θ ;on the space V λ corresponding to the eigenvalue λ = l(l + 1), we have P g (ω) Vλ = P r (ω)+λ. Therefore, the only problem is to show the radial Lemma 1.5 in this case, which is in fact no simpler than the general case. (Note that we take a different path here than [41] and [10], using only real microlocal analysis near the trapped set, which immediately gives polynomial resolvent bounds). 2. Preliminaries 2.1. Pseudodifferential Operators and Microlocalization First of all, we review the asses of semiassical pseudodifferential operators on manifolds and introduce notation used for these asses; see [54, Sections 9.3 and 14.2] or [21] for more information. For k R, we consider the symbol ass S k (R n ) consisting of functions a(x, ξ; h) smooth in (x, ξ) R 2n and satisfying the following growth conditions: for each compact set K R n and each pair of multi-indices α, β, there exists a constant C αβk such that α x β ξ a(x, ξ; h) C αβk ξ k β, x K, ξ R n,h>0. If we treat R 2n as the cotangent bundle to R n, then the ass S k is invariant under changes of variables; this makes it possible, given a manifold M, to define the ass S k (M) of symbols depending on (x, ξ) T M.
15 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter 1115 If k R and a j (x, ξ) S k j (M), j =0, 1,..., is a sequence of symbols, then there exists the asymptotic sum a(x, ξ; h) j 0 h j a j (x, ξ); (2.1) i.e., a symbol a(x, ξ; h) S k (M) such that for every J =0, 1,..., a(x, ξ; h) h j a j (x, ξ) h J S k J. 0 j<j The asymptotic sum a is unique modulo the ass h S of symbols all of whose derivatives decay faster than h N ξ N for each N on any compact set in x. Ifa is given by an asymptotic sum of the form (2.1), then we call it a assical symbol and write a S k (M). We call a 0(x, ξ) the principal part of the symbol a(x, ξ; h). Let Ψ k (M) be the algebra of (properly supported) semiassical pseudodifferential operators on M with symbols in S k (M). If Hh,loc m (M), m R, consists of functions locally lying in the semiassical Sobolev space, then every element of Ψ k (M) is continuous Hh,loc m (M) Hm k h,loc (M) with every operator seminorm being O(1) as h 0. Let Ψ k (M) be the algebra of operators with symbols in S k (M) and Ψ (M) be the union of Ψ k for all k. Next, let the operator ass h Ψ (M) correspond to the symbol ass h S (M); it can be characterized as follows: A h Ψ (M) if and only if for each N, A is continuous H N h,loc (M) HN h,loc (M), with every operator seminorm being O(h N ). The full symbol of an element of Ψ k (M) cannot be recovered as a function on T M; however, if A Ψ k (M), then the principal symbol of A is an invariantly defined function on the cotangent bundle. If M is an open subset of R n, then we can define the full symbol of a pseudodifferential operator modulo h S ; we will always use Weyl quantization. We now introduce microlocalization; see also [54, Section 8.4] and [44, Section 3]. Define U T M to be conic at infinity, if there exists a conic set V T M such that the symmetric difference of U and V is bounded when restricted to every compact subset of M. Fora S k (M) andu T M open and conic at infinity, we say that a is rapidly decaying on U, if for every V U osed in T M, conic at infinity, and with compact projection onto M, every derivative of a decays on V faster than h N ξ N for every N. We say that A Ψ k (M) vanishes microlocally on U if its full symbol (in any coordinate system) is rapidly decaying on U. IfA, B Ψ k (M), then we say that A = B microlocally on U, ifa B vanishes microlocally on U. For A Ψ k (M), we define the semiassical wavefront set WF h (A) T M as follows: (x, ξ) WF h (A) if and only if A vanishes microlocally on some neighborhood of (x, ξ). The set WF h (A) is osed; however, it need not be conic at infinity. Next, we say that A is compactly microlocalized, if there exists a compact set K T M such that A vanishes microlocally on T M \K. We denote by Ψ comp (M) the set of compactly microlocalized operators. Here are some properties of microlocalization:
16 1116 S. Dyatlov Ann. Henri Poincaré If A vanishes microlocally on U 1 and U 2, then it vanishes microlocally on U 1 U 2. The set of pseudodifferential operators vanishing microlocally on some open and conic at infinity U T M is a two-sided ideal; so is the set of operators with wavefront set contained in some osed V T M.In particular, WF h (AB) WF h (A) WF h (B). A vanishes microlocally on the whole T M if and only if it lies in h Ψ. If A vanishes microlocally on U, then WF h (A) U = ; the converse is true if U is bounded. However, 7 A does not necessarily vanish microlocally on the complement of WF h (A); for example, the operator A = e 1/h lies in Ψ 0 and has an empty wavefront set, yet it does not lie in h Ψ. The set Ψ comp forms a two-sided ideal and it lies in Ψ N for every N. Each A Ψ comp vanishes microlocally on the complement of WF h (A). Let A Ψ k (M) and let its symbol in some coordinate system have the form (2.1); introduce V = j 0 supp a j. Then, A vanishes microlocally on some open set U if and only if U V = ; A is compactly microlocalized if V is bounded, and WF h (A) is the osure of V. We now consider microlocally defined operators. Let U T M be open. A local pseudodifferential operator A on U is, by definition, a map B [A B] Ψ comp (M)/h Ψ (M), B Ψ comp (M), WF h (B) U, such that: WF h ([A B]) WF h (B). If B 1,B 2 Ψ comp (M) andwf h (B j ) U, then [A (B 1 + B 2 )] = [A B 1 ]+[A B 2 ]. If C Ψ k (M), then [A B]C =[A (BC)]. We denote by Ψ loc (U) the set of all local operators on U. Note that a local operator is only defined modulo an h Ψ remainder. If A Ψ k (M), then the corresponding local operator à is given by [à B] =AB mod h Ψ ; we say that A represents Ã. ForM = Rn and U T M, there is a one-to-one correspondence between local operators and their full symbols modulo h ; the symbols of local operators are functions a(x, ξ; h) smooth in (x, ξ) U all of whose derivatives are uniformly bounded in h on compact subsets of U. In fact, for a symbol a(x, ξ; h), the corresponding local operator is defined by [A B] =(a#b) w (x, hd x ), where b(x, ξ; h) is the full symbol of B Ψ comp ; since b is compactly supported inside U modulo O(h )anda is defined on U, we can define the symbol product a#b uniquely modulo O(h ). In particular, a assical local operator A Ψ loc (M) is uniquely determined by the terms of 7 This issue can be avoided if we consider WF h (A) as a subset of the fiber compactified cotangent bundle T M,asin[50, Section 2.1]. Then, an operator is compactly microlocalized if and only if its wavefront set does not intersect the fiber infinity.
17 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter 1117 the decomposition (2.1) of its full symbol. Note that U is not required to be conic at infinity, and we do not impose any conditions on the growth of a as ξ. Local operators form a sheaf of algebras; that is, one can multiply local operators defined on the same set, restrict a local operator to a smaller set, and reconstruct a local operator from its restrictions to members of some finite open covering of U. This makes it possible to describe any local operator A Ψ loc (U) on a manifold using its full symbols in various coordinate charts. For A Ψ loc (U), one can define its wavefront set WF h (A) as follows: (x 0,ξ 0 ) WF h (A) if and only if the full symbol of A is O(h ) in some neighborhood of (x 0,ξ 0 ). If A Ψ k represents à Ψloc, then WF h (A) =WF h (Ã); in general, wavefront sets of local operators obey WF h (A + B) WF h (A) WF h (B) and WF h (AB) WF h (A) WF h (B). Finally, we study microlocalization of arbitrary operators. Let M 1 and M 2 be two manifolds. An h-dependent family of (properly supported) operators A(h) :C (M 1 ) D (M 2 ) is called tempered, or polynomially bounded, if for every compact K 1 M 1, there exist N and C such that for any u C0 (K 1 ), A(h)u H N h (M2) Ch N u H N h. Note that the composition of a tempered operator with an element of Ψ k is still tempered. We can also treat distributions on M 2 as operators from a singleton to M 2. For a tempered family A(h), we define its wavefront set WF h (A) T (M 1 M 2 ) as follows: (x, ξ; y, η) WF h (A), if and only if there exist neighborhoods U 1 (x, ξ) andu 2 (y, η) such that for every B j Ψ comp (M j ) with WF h (B j ) U j,wehaveb 2 A(h)B 1 h Ψ. We say that A 1 = A 2 microlocally in some open and bounded U T M,ifWF h (A 1 A 2 ) U =. Also, A(h) is said to be compactly microlocalized, if there exist C j Ψ comp (M j ) such that A(h) C 2 A(h)C 1 h Ψ. In this case, all operator norms A(h) N H 1 h HN 2 are equivalent modulo O(h ); if any of these norms is O(h r ) h for some constant r, we write A(h) = O(h r ). Here are some properties: If A is compactly microlocalized, then WF h (A) is compact. The converse, however, need not be true. If A Ψ k (M), then the two definitions of compact microlocalization of A (via its symbol and as a tempered family of operators) agree; the wavefront set of A as a tempered family of operators is just {(x, ξ; x, ξ) (x, ξ) WF h (A)}. If A 1,A 2 are two tempered operators and at least one of them is either compactly microlocalized or pseudodifferential, then the product A 2 A 1 is a tempered operator, and WF h (A 2 A 1 ) WF h (A 1 ) WF h (A 2 ) = {(x, ξ; z,ζ) (y, η) :(x, ξ; y, η) WF h (A 1 ), (y, η; z,ζ) WF h (A 2 )}. Moreover, if both A 1,A 2 are compactly microlocalized, so is A 2 A 1. Let us quote the following microlocalization fact for oscillatory integrals, which is the starting point for the construction of semiassical Fourier integral operators used in Sect. 2.3:
18 1118 S. Dyatlov Ann. Henri Poincaré Proposition 2.1. Assume that M is a manifold, U M R m is open, ϕ(x, θ) is a smooth real-valued function on U, withx M and θ R m,anda(x, θ) C0 (U). Then, the family of distributions u(x) = e iϕ(x,θ)/h a(x, θ)dθ R m is compactly microlocalized and WF h (u) {(x, x ϕ(x, θ)) (x, θ) supp a, θ ϕ(x, θ) =0} Ellipticity and Formal Functional Calculus Assume that U T M is open and A Ψ loc (U). We say that A is (semiassically) elliptic on U if its principal symbol does not vanish on U. Under this condition, there exists unique operator A 1 Ψ loc (U) such that A 1 A, AA 1 = I as local operators. The next proposition provides the form of the symbol of A 1 ; it is based on the standard parametrix construction: Proposition 2.2. Fix a coordinate system on M. Assume that A Ψ loc (U) is elliptic and has the full symbol a a 0 + ha 1 + h 2 a 2 +.Then,A 1 has the full symbol b b 0 + hb 1 + h 2 b 2 +,whereeachb j is a linear combination with constant coefficients of the terms of the form a M 1 0 M m=1 Here the (multi)indices α m,β m,l m satisfy the condition M M M α m = β m = j l m. m=1 m=1 x αm βm ξ a lm. (2.2) m=1 Furthermore, we can assume that α m + β m + l m > 0 for all m. Proof. We call (2.2) an expression of type (M α,m β,l), where M α,m β,l are the sums of α m, β m,andl m, respectively. If f is an expression of type (M α,m β,l), then we can prove by induction that x α β ξ f is an expression of type (M α + α,m β + β,l). Now, we write the equation a#b = 1; the principal term gives b 0 = a 1 0, and the next terms give that each b j is the sum of expressions of type (j L, j L, L), by induction and the formula for the symbol product a#b. Let A Ψ k ; we say that it is elliptic on an open conic at infinity U T M in the ass Ψ k (or microlocally elliptic), if its principal symbol a 0 satisfies a 0 (x, ξ) ξ k /C(K) for(x, ξ) in any given osed conic at infinity K U with compact projection onto M, and some constant C(K) depending on K. In this case, the full symbol of A 1 satisfies the decay conditions of the ass Ψ k in U. In particular, if A Ψ k is elliptic in the ass Ψk everywhere, then we can define A 1 Ψ k for h small enough. We now construct functional calculus of local real principal pseudodifferential operators. For this, we use holomorphic functional calculus
19 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter 1119 [25, Section 7.3]; another approach would be via almost analytic continuation [21, Chapter 8]. First, assume that A Ψ comp (M) has compactly supported Schwartz kernel. In particular, the principal symbol a 0 of A is compactly supported; let K C be the image of a 0.Letf(z) be holomorphic in a neighborhood Ω of K, and let γ Ω be a contour such that K lies inside of γ. For each h, the operator A is bounded L 2 L 2 ;forh small enough, its spectrum lies inside of γ. Then, we can define the operator f(a) by the formula f(a) = 1 f(z)(z A) 1 dz. 2πi γ For z γ, the operator z A is elliptic in the ass Ψ 0 ; therefore, (z A) 1 Ψ 0 (M). It follows that f(a) Ψ0 (M). By Proposition 2.2, the full symbol of f(a) (in any coordinate system) is the asymptotic sum j=0 h j 2j M=0 f (M) (a 0 (x, ξ))b jm (x, ξ). (2.3) Here a a 0 + ha 1 + is the full symbol of A; b jm are the functions resulting from applying certain nonlinear differential operators to a 0,a 1,... Now, assume that U T M is open and A Ψ loc (U) has real-valued principal symbol a 0. Then, the formula (2.3) can be used to define an operator f[a] Ψ loc (U) for any f C (R). Note that the principal symbol of (z A) 1 is (z a 0 ) 1 ; therefore, the principal symbol of f[a] isf a 0.The constructed operation possesses the following properties of functional calculus: Proposition 2.3. Assume that U T M is open, A Ψ loc (U), andf,g C (R). Then: 1. WF h (f[a]) a 1 0 (supp f), wherea 0 is the principal symbol of A. 2. If f(t) = K j=0 f jt j is a polynomial, then f[a] =f(a), wheref(a) = j f ja j. 3. (f + g)[a] =f[a]+g[a] and (fg)[a] =f[a]g[a]. 4. If B Ψ loc (U) and [A, B] =0, then [f[a],b]=0. The identities in parts 2 4 are equalities of local operators; in particular, they inude the h Ψ error. In fact, the operator f[a] is only defined uniquely modulo h Ψ. Proof. 1. Follows immediately from (2.3). 2. Take an open set V compactly contained in U; then, there exists Ã Ψ comp (M) such that A = à microlocally on V. Since f is entire and à is compactly microlocalized, we can define f(ã) by means of holomorphic functional calculus; it is be a pseudodifferential operator representing f[ã]. Now, f(a) =f(ã) microlocally on V by properties of multiplication of pseudodifferential operators and f[a] =f[ã] microlocally on V by (2.3); therefore, f(a) =f[a] microlocally on V. Since V was arbitrary, we have f(a) =f[a] microlocally on the whole U.
20 1120 S. Dyatlov Ann. Henri Poincaré 3. We only prove the second statement. It suffices to show that for every coordinate system on M, the full symbols of (fg)[a] andf[a]g[a] are equal. However, the terms in the asymptotic decomposition of the full symbol of (fg)[a] f[a]g[a] at(x, ξ) only depend on the derivatives of the full symbol of A at (x, ξ) and the derivatives of f and g at a 0 (x, ξ). Therefore, it suffices to consider the case when f and g are polynomials. In this case, we can use the previous part of the proposition and the fact that f(a)g(a) =(fg)(a). 4. This is proven similarly to the previous part, using the fact that [A, B] =0 yields [f(a),b] = 0 for every polynomial f. Finally, under certain conditions on the growth of f and the symbol of A at infinity, f[a] is a globally defined operator: Proposition 2.4. Assume that A Ψ k (M), withk 0, and that A is elliptic in the ass Ψ k outside of a compact subset of T M. Also, assume that f C (R) is a symbol of order s, in the sense that for each l, there exists a constant C l such that f (l) (t) C l t s l, t R. Then, f[a] is represented by an operator in Ψ sk (M). Proof. We use (2.3); by Proposition 2.2, the symbol b jm lies in S km j. Since f is a symbol of order s, f (M) is a symbol of order s M. Then, since a 0 S k is elliptic outside of a compact set and k 0, we have f (M) a 0 S k(s M). It follows that each term in (2.3) lies in S sk j ; therefore, this asymptotic sum gives an element of Ψ sk Quantizing Canonical Transformations Assume that M 1 and M 2 are two manifolds of the same dimension. Recall that the symplectic form ωj S on T M j is given by ωj S = dσs j, where σs j = ξdx is the canonical 1-form. We let K j T M j be compact and assume that Φ:T M 1 T M 2 is a symplectomorphism defined in a neighborhood of K 1 and such that Φ(K 1 )=K 2. Then, the form σ1 S Φ σ2 S is osed; we say that Φ is an exact symplectomorphism if this form is exact. Define the assical action over a osed curve in T M j as the integral of σj S over this curve; then, Φ is exact if and only if for each osed curve γ in the domain of Φ, the assical action over γ is equal to the assical action over Φ γ. We can quantize exact symplectomorphisms as follows: Proposition 2.5. Assume that Φ is an exact symplectomorphism. Then, there exist h-dependent families of operators B 1 : D (M 1 ) C0 (M 2 ), B 2 : D (M 2 ) C0 (M 1 ) such that: 1. Each B j is compactly microlocalized and has operator norm O(1); moreover, WF h (B 1 ) is contained in the graph of Φ and WF h (B 2 ) is contained in the graph of Φ 1.
21 Vol. 13 (2012) Asymptotic Distribution of QNMs for Kerr de Sitter The operators B 1 B 2 and B 2 B 1 are equal to the identity microlocally near K 2 K 2 and K 1 K 1, respectively. 3. For each P Ψ (M 1 ), there exists Q Ψ (M 2 ) that is intertwined with P via B 1 and B 2 : B 1 P = QB 1, PB 2 = B 2 Q microlocally near K 1 K 2 and K 2 K 1, respectively. Similarly, for each Q Ψ (M 2 ) there exists P Ψ (M 1 ) intertwined with it. Finally, if P and Q are intertwined via B 1 and B 2 and p and q are their principal symbols, then p = q Φ near K 1. If the properties 1 3 hold, we say that the pair (B 1,B 2 ) quantizes the canonical transformation Φ near K 1 K 2. Proof. We take B 1,B 2 to be semiassical Fourier integral operators associated with Φ and Φ 1, respectively; their symbols are taken compactly supported and elliptic in a neighborhood of K 1 K 2. The existence of globally defined elliptic symbols follows from the exactness of Φ; the rest follows from calculus of Fourier integral operators. See [30, Chapter 8] or [51, Chapter 2] for more details. Note that the operators B 1 and B 2 quantizing a given canonical transformation are not unique. In fact, if X j Ψ comp (M j ) are elliptic near K j and Y j Ψ comp (M j ) are their inverses near K j, then (X 2 B 1 X 1,Y 1 B 2 Y 2 ) also quantizes Φ; moreover, P is intertwined with Q via the new pair of operators if and only if X 1 PY 1 is intertwined with Y 2 QX 2 via (B 1,B 2 ). We now study microlocal properties of Schrödinger propagators. Take A Ψ comp (M) with compactly supported Schwartz kernel and let a 0 be its principal symbol; we assume that a 0 is real-valued. In this case the Hamiltonian flow exp(th a0 ), t R, is a family of symplectomorphisms defined on the whole T M; it is the identity outside of supp a 0. Moreover, exp(th a0 ) is exact; indeed, if V = H a0, then by Cartan s formula L V σ S = d(i V σ S )+i V d(σ S )=d(i V σ S a 0 ) is exact. Therefore, d t exp(tv ) σ S =exp(tv ) L V σ S is exact and exp(tv ) σ S σ S is exact for all t. For each t, define the operator exp(ita/h) as the solution to the Schrödinger equation hd t exp(ita/h) =A exp(ita/h) = exp(ita/h)a in the algebra of bounded operators on L 2 (M), with the initial condition exp(i0a/h) =I. Such a family exists since A is a bounded operator on L 2 (M) for all h. Here are some of its properties (see also [54, Chapter 10]): Proposition The operator exp(ita/h) I is compactly microlocalized and has operator norm O(1).
Unit Relational Algebra 1 1. Relational Algebra 1. Unit 3.3
 Relational Algebra 1 Unit 3.3 Unit 3.3 - Relational Algebra 1 1 Relational Algebra Relational Algebra is : the formal description of how a relational database operates the mathematics which underpin SQL
Relational Algebra 1 Unit 3.3 Unit 3.3 - Relational Algebra 1 1 Relational Algebra Relational Algebra is : the formal description of how a relational database operates the mathematics which underpin SQL
Slope-Intercept Formula
 LESSON 7 Slope Intercept Formula LESSON 7 Slope-Intercept Formula Here are two new words that describe lines slope and intercept. The slope is given by m (a mountain has slope and starts with m), and intercept
LESSON 7 Slope Intercept Formula LESSON 7 Slope-Intercept Formula Here are two new words that describe lines slope and intercept. The slope is given by m (a mountain has slope and starts with m), and intercept
Physical origin of the Gouy phase shift by Simin Feng, Herbert G. Winful Opt. Lett. 26, (2001)
 by Simin Feng, Herbert G. Winful Opt. Lett. 26, 485-487 (2001) http://smos.sogang.ac.r April 18, 2014 Introduction What is the Gouy phase shift? For Gaussian beam or TEM 00 mode, ( w 0 r 2 E(r, z) = E
by Simin Feng, Herbert G. Winful Opt. Lett. 26, 485-487 (2001) http://smos.sogang.ac.r April 18, 2014 Introduction What is the Gouy phase shift? For Gaussian beam or TEM 00 mode, ( w 0 r 2 E(r, z) = E
UNIVERSITETET I OSLO
 UNIVERSITETET I OSLO Det matematisk-naturvitenskapelige fakultet Eksamen i MAT2400 Analyse 1. Eksamensdag: Onsdag 15. juni 2011. Tid for eksamen: 09.00 13.00 Oppgavesettet er på 6 sider. Vedlegg: Tillatte
UNIVERSITETET I OSLO Det matematisk-naturvitenskapelige fakultet Eksamen i MAT2400 Analyse 1. Eksamensdag: Onsdag 15. juni 2011. Tid for eksamen: 09.00 13.00 Oppgavesettet er på 6 sider. Vedlegg: Tillatte
Graphs similar to strongly regular graphs
 Joint work with Martin Ma aj 5th June 2014 Degree/diameter problem Denition The degree/diameter problem is the problem of nding the largest possible graph with given diameter d and given maximum degree
Joint work with Martin Ma aj 5th June 2014 Degree/diameter problem Denition The degree/diameter problem is the problem of nding the largest possible graph with given diameter d and given maximum degree
Databases 1. Extended Relational Algebra
 Databases 1 Extended Relational Algebra Relational Algebra What is an Algebra? Mathematical system consisting of: Operands --- variables or values from which new values can be constructed. Operators ---
Databases 1 Extended Relational Algebra Relational Algebra What is an Algebra? Mathematical system consisting of: Operands --- variables or values from which new values can be constructed. Operators ---
UNIVERSITY OF OSLO DEPARTMENT OF ECONOMICS
 UNIVERSITY OF OSLO DEPARTMENT OF ECONOMICS Postponed exam: ECON420 Mathematics 2: Calculus and linear algebra Date of exam: Tuesday, June 8, 203 Time for exam: 09:00 a.m. 2:00 noon The problem set covers
UNIVERSITY OF OSLO DEPARTMENT OF ECONOMICS Postponed exam: ECON420 Mathematics 2: Calculus and linear algebra Date of exam: Tuesday, June 8, 203 Time for exam: 09:00 a.m. 2:00 noon The problem set covers
Moving Objects. We need to move our objects in 3D space.
 Transformations Moving Objects We need to move our objects in 3D space. Moving Objects We need to move our objects in 3D space. An object/model (box, car, building, character,... ) is defined in one position
Transformations Moving Objects We need to move our objects in 3D space. Moving Objects We need to move our objects in 3D space. An object/model (box, car, building, character,... ) is defined in one position
Neural Network. Sensors Sorter
 CSC 302 1.5 Neural Networks Simple Neural Nets for Pattern Recognition 1 Apple-Banana Sorter Neural Network Sensors Sorter Apples Bananas 2 Prototype Vectors Measurement vector p = [shape, texture, weight]
CSC 302 1.5 Neural Networks Simple Neural Nets for Pattern Recognition 1 Apple-Banana Sorter Neural Network Sensors Sorter Apples Bananas 2 Prototype Vectors Measurement vector p = [shape, texture, weight]
Universitetet i Bergen Det matematisk-naturvitenskapelige fakultet Eksamen i emnet Mat131 - Differensiallikningar I Onsdag 25. mai 2016, kl.
 1 MAT131 Bokmål Universitetet i Bergen Det matematisk-naturvitenskapelige fakultet Eksamen i emnet Mat131 - Differensiallikningar I Onsdag 25. mai 2016, kl. 09-14 Oppgavesettet er 4 oppgaver fordelt på
1 MAT131 Bokmål Universitetet i Bergen Det matematisk-naturvitenskapelige fakultet Eksamen i emnet Mat131 - Differensiallikningar I Onsdag 25. mai 2016, kl. 09-14 Oppgavesettet er 4 oppgaver fordelt på
Gradient. Masahiro Yamamoto. last update on February 29, 2012 (1) (2) (3) (4) (5)
 Gradient Masahiro Yamamoto last update on February 9, 0 definition of grad The gradient of the scalar function φr) is defined by gradφ = φr) = i φ x + j φ y + k φ ) φ= φ=0 ) ) 3) 4) 5) uphill contour downhill
Gradient Masahiro Yamamoto last update on February 9, 0 definition of grad The gradient of the scalar function φr) is defined by gradφ = φr) = i φ x + j φ y + k φ ) φ= φ=0 ) ) 3) 4) 5) uphill contour downhill
Solutions #12 ( M. y 3 + cos(x) ) dx + ( sin(y) + z 2) dy + xdz = 3π 4. The surface M is parametrized by σ : [0, 1] [0, 2π] R 3 with.
![Solutions #12 ( M. y 3 + cos(x) ) dx + ( sin(y) + z 2) dy + xdz = 3π 4. The surface M is parametrized by σ : [0, 1] [0, 2π] R 3 with. Solutions #12 ( M. y 3 + cos(x) ) dx + ( sin(y) + z 2) dy + xdz = 3π 4. The surface M is parametrized by σ : [0, 1] [0, 2π] R 3 with.](/thumbs/80/81672304.jpg) Solutions #1 1. a Show that the path γ : [, π] R 3 defined by γt : cost ı sint j sint k lies on the surface z xy. b valuate y 3 cosx dx siny z dy xdz where is the closed curve parametrized by γ. Solution.
Solutions #1 1. a Show that the path γ : [, π] R 3 defined by γt : cost ı sint j sint k lies on the surface z xy. b valuate y 3 cosx dx siny z dy xdz where is the closed curve parametrized by γ. Solution.
TFY4170 Fysikk 2 Justin Wells
 TFY4170 Fysikk 2 Justin Wells Forelesning 5: Wave Physics Interference, Diffraction, Young s double slit, many slits. Mansfield & O Sullivan: 12.6, 12.7, 19.4,19.5 Waves! Wave phenomena! Wave equation
TFY4170 Fysikk 2 Justin Wells Forelesning 5: Wave Physics Interference, Diffraction, Young s double slit, many slits. Mansfield & O Sullivan: 12.6, 12.7, 19.4,19.5 Waves! Wave phenomena! Wave equation
Dynamic Programming Longest Common Subsequence. Class 27
 Dynamic Programming Longest Common Subsequence Class 27 Protein a protein is a complex molecule composed of long single-strand chains of amino acid molecules there are 20 amino acids that make up proteins
Dynamic Programming Longest Common Subsequence Class 27 Protein a protein is a complex molecule composed of long single-strand chains of amino acid molecules there are 20 amino acids that make up proteins
Mathematics 114Q Integration Practice Problems SOLUTIONS. = 1 8 (x2 +5x) 8 + C. [u = x 2 +5x] = 1 11 (3 x)11 + C. [u =3 x] = 2 (7x + 9)3/2
![Mathematics 114Q Integration Practice Problems SOLUTIONS. = 1 8 (x2 +5x) 8 + C. [u = x 2 +5x] = 1 11 (3 x)11 + C. [u =3 x] = 2 (7x + 9)3/2 Mathematics 114Q Integration Practice Problems SOLUTIONS. = 1 8 (x2 +5x) 8 + C. [u = x 2 +5x] = 1 11 (3 x)11 + C. [u =3 x] = 2 (7x + 9)3/2](/thumbs/77/75859899.jpg) Mathematics 4Q Name: SOLUTIONS. (x + 5)(x +5x) 7 8 (x +5x) 8 + C [u x +5x]. (3 x) (3 x) + C [u 3 x] 3. 7x +9 (7x + 9)3/ [u 7x + 9] 4. x 3 ( + x 4 ) /3 3 8 ( + x4 ) /3 + C [u + x 4 ] 5. e 5x+ 5 e5x+ + C
Mathematics 4Q Name: SOLUTIONS. (x + 5)(x +5x) 7 8 (x +5x) 8 + C [u x +5x]. (3 x) (3 x) + C [u 3 x] 3. 7x +9 (7x + 9)3/ [u 7x + 9] 4. x 3 ( + x 4 ) /3 3 8 ( + x4 ) /3 + C [u + x 4 ] 5. e 5x+ 5 e5x+ + C
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Utsatt ksamen i: ECON3120/4120 Matematikk 2: Matematisk analyse og lineær algebra Postponed exam: ECON3120/4120 Mathematics 2: Calculus and linear algebra Eksamensdag:
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Utsatt ksamen i: ECON3120/4120 Matematikk 2: Matematisk analyse og lineær algebra Postponed exam: ECON3120/4120 Mathematics 2: Calculus and linear algebra Eksamensdag:
Trigonometric Substitution
 Trigonometric Substitution Alvin Lin Calculus II: August 06 - December 06 Trigonometric Substitution sin 4 (x) cos (x) dx When you have a product of sin and cos of different powers, you have three different
Trigonometric Substitution Alvin Lin Calculus II: August 06 - December 06 Trigonometric Substitution sin 4 (x) cos (x) dx When you have a product of sin and cos of different powers, you have three different
Second Order ODE's (2P) Young Won Lim 7/1/14
 Second Order ODE's (2P) Copyright (c) 2011-2014 Young W. Lim. Permission is granted to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or
Second Order ODE's (2P) Copyright (c) 2011-2014 Young W. Lim. Permission is granted to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or
Call function of two parameters
 Call function of two parameters APPLYUSER USER x fµ 1 x 2 eµ x 1 x 2 distinct e 1 0 0 v 1 1 1 e 2 1 1 v 2 2 2 2 e x 1 v 1 x 2 v 2 v APPLY f e 1 e 2 0 v 2 0 µ Evaluating function application The math demands
Call function of two parameters APPLYUSER USER x fµ 1 x 2 eµ x 1 x 2 distinct e 1 0 0 v 1 1 1 e 2 1 1 v 2 2 2 2 e x 1 v 1 x 2 v 2 v APPLY f e 1 e 2 0 v 2 0 µ Evaluating function application The math demands
Splitting the differential Riccati equation
 Splitting the differential Riccati equation Tony Stillfjord Numerical Analysis, Lund University Joint work with Eskil Hansen Innsbruck Okt 15, 2014 Outline Splitting methods for evolution equations The
Splitting the differential Riccati equation Tony Stillfjord Numerical Analysis, Lund University Joint work with Eskil Hansen Innsbruck Okt 15, 2014 Outline Splitting methods for evolution equations The
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Tirsdag 7. juni
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Tirsdag 7. juni
5 E Lesson: Solving Monohybrid Punnett Squares with Coding
 5 E Lesson: Solving Monohybrid Punnett Squares with Coding Genetics Fill in the Brown colour Blank Options Hair texture A field of biology that studies heredity, or the passing of traits from parents to
5 E Lesson: Solving Monohybrid Punnett Squares with Coding Genetics Fill in the Brown colour Blank Options Hair texture A field of biology that studies heredity, or the passing of traits from parents to
Stationary Phase Monte Carlo Methods
 Stationary Phase Monte Carlo Methods Daniel Doro Ferrante G. S. Guralnik, J. D. Doll and D. Sabo HET Physics Dept, Brown University, USA. danieldf@het.brown.edu www.het.brown.edu Introduction: Motivations
Stationary Phase Monte Carlo Methods Daniel Doro Ferrante G. S. Guralnik, J. D. Doll and D. Sabo HET Physics Dept, Brown University, USA. danieldf@het.brown.edu www.het.brown.edu Introduction: Motivations
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Date of exam: Friday, May
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Date of exam: Friday, May
Speed Racer Theme. Theme Music: Cartoon: Charles Schultz / Jef Mallett Peanuts / Frazz. September 9, 2011 Physics 131 Prof. E. F.
 September 9, 2011 Physics 131 Prof. E. F. Redish Theme Music: Speed Racer Theme Cartoon: Charles Schultz / Jef Mallett Peanuts / Frazz 1 Reading questions Are the lines on the spatial graphs representing
September 9, 2011 Physics 131 Prof. E. F. Redish Theme Music: Speed Racer Theme Cartoon: Charles Schultz / Jef Mallett Peanuts / Frazz 1 Reading questions Are the lines on the spatial graphs representing
Generalization of age-structured models in theory and practice
 Generalization of age-structured models in theory and practice Stein Ivar Steinshamn, stein.steinshamn@snf.no 25.10.11 www.snf.no Outline How age-structured models can be generalized. What this generalization
Generalization of age-structured models in theory and practice Stein Ivar Steinshamn, stein.steinshamn@snf.no 25.10.11 www.snf.no Outline How age-structured models can be generalized. What this generalization
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON20/420 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON20/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Fredag 2. mai
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON20/420 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON20/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Fredag 2. mai
Maple Basics. K. Cooper
 Basics K. Cooper 2012 History History 1982 Macsyma/MIT 1988 Mathematica/Wolfram 1988 /Waterloo Others later History Why? Prevent silly mistakes Time Complexity Plots Generate LATEX This is the 21st century;
Basics K. Cooper 2012 History History 1982 Macsyma/MIT 1988 Mathematica/Wolfram 1988 /Waterloo Others later History Why? Prevent silly mistakes Time Complexity Plots Generate LATEX This is the 21st century;
Existence of resistance forms in some (non self-similar) fractal spaces
 Existence of resistance forms in some (non self-similar) fractal spaces Patricia Alonso Ruiz D. Kelleher, A. Teplyaev University of Ulm Cornell, 12 June 2014 Motivation X Fractal Motivation X Fractal Laplacian
Existence of resistance forms in some (non self-similar) fractal spaces Patricia Alonso Ruiz D. Kelleher, A. Teplyaev University of Ulm Cornell, 12 June 2014 Motivation X Fractal Motivation X Fractal Laplacian
SVM and Complementary Slackness
 SVM and Complementary Slackness David Rosenberg New York University February 21, 2017 David Rosenberg (New York University) DS-GA 1003 February 21, 2017 1 / 20 SVM Review: Primal and Dual Formulations
SVM and Complementary Slackness David Rosenberg New York University February 21, 2017 David Rosenberg (New York University) DS-GA 1003 February 21, 2017 1 / 20 SVM Review: Primal and Dual Formulations
Ringvorlesung Biophysik 2016
 Ringvorlesung Biophysik 2016 Born-Oppenheimer Approximation & Beyond Irene Burghardt (burghardt@chemie.uni-frankfurt.de) http://www.theochem.uni-frankfurt.de/teaching/ 1 Starting point: the molecular Hamiltonian
Ringvorlesung Biophysik 2016 Born-Oppenheimer Approximation & Beyond Irene Burghardt (burghardt@chemie.uni-frankfurt.de) http://www.theochem.uni-frankfurt.de/teaching/ 1 Starting point: the molecular Hamiltonian
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON3120/4120 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON3120/4120 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Tirsdag
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON3120/4120 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON3120/4120 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Tirsdag
Oppgave 1. ( xφ) φ x t, hvis t er substituerbar for x i φ.
 Oppgave 1 Beviskalklen i læreboka inneholder sluttningsregelen QR: {ψ φ}, ψ ( xφ). En betingelse for å anvende regelen er at det ikke finnes frie forekomste av x i ψ. Videre så inneholder beviskalklen
Oppgave 1 Beviskalklen i læreboka inneholder sluttningsregelen QR: {ψ φ}, ψ ( xφ). En betingelse for å anvende regelen er at det ikke finnes frie forekomste av x i ψ. Videre så inneholder beviskalklen
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON30/40 Matematikk : Matematisk analyse og lineær algebra Exam: ECON30/40 Mathematics : Calculus and Linear Algebra Eksamensdag: Tirsdag 0. desember
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON30/40 Matematikk : Matematisk analyse og lineær algebra Exam: ECON30/40 Mathematics : Calculus and Linear Algebra Eksamensdag: Tirsdag 0. desember
UNIVERSITETET I OSLO
 UNIVERSITETET I OSLO Det matematisk-naturvitenskapelige fakultet Eksamen i INF 3230 Formell modellering og analyse av kommuniserende systemer Eksamensdag: 4. juni 2010 Tid for eksamen: 9.00 12.00 Oppgavesettet
UNIVERSITETET I OSLO Det matematisk-naturvitenskapelige fakultet Eksamen i INF 3230 Formell modellering og analyse av kommuniserende systemer Eksamensdag: 4. juni 2010 Tid for eksamen: 9.00 12.00 Oppgavesettet
Qi-Wu-Zhang model. 2D Chern insulator. León Martin. 19. November 2015
 Qi-Wu-Zhang model 2D Chern insulator León Martin 19. November 2015 Motivation Repeat: Rice-Mele-model Bulk behavior Edge states Layering 2D Chern insulators Robustness of edge states Motivation topological
Qi-Wu-Zhang model 2D Chern insulator León Martin 19. November 2015 Motivation Repeat: Rice-Mele-model Bulk behavior Edge states Layering 2D Chern insulators Robustness of edge states Motivation topological
Level Set methods. Sandra Allaart-Bruin. Level Set methods p.1/24
 Level Set methods Sandra Allaart-Bruin sbruin@win.tue.nl Level Set methods p.1/24 Overview Introduction Level Set methods p.2/24 Overview Introduction Boundary Value Formulation Level Set methods p.2/24
Level Set methods Sandra Allaart-Bruin sbruin@win.tue.nl Level Set methods p.1/24 Overview Introduction Level Set methods p.2/24 Overview Introduction Boundary Value Formulation Level Set methods p.2/24
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Onsdag 6. desember
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Onsdag 6. desember
Trust region methods: global/local convergence, approximate January methods 24, / 15
 Trust region methods: global/local convergence, approximate methods January 24, 2014 Trust region methods: global/local convergence, approximate January methods 24, 2014 1 / 15 Trust-region idea Model
Trust region methods: global/local convergence, approximate methods January 24, 2014 Trust region methods: global/local convergence, approximate January methods 24, 2014 1 / 15 Trust-region idea Model
Kneser hypergraphs. May 21th, CERMICS, Optimisation et Systèmes
 Kneser hypergraphs Frédéric Meunier May 21th, 2015 CERMICS, Optimisation et Systèmes Kneser hypergraphs m, l, r three integers s.t. m rl. Kneser hypergraph KG r (m, l): V (KG r (m, l)) = ( [m]) l { E(KG
Kneser hypergraphs Frédéric Meunier May 21th, 2015 CERMICS, Optimisation et Systèmes Kneser hypergraphs m, l, r three integers s.t. m rl. Kneser hypergraph KG r (m, l): V (KG r (m, l)) = ( [m]) l { E(KG
On time splitting for NLS in the semiclassical regime
 On time splitting for NLS in the semiclassical regime Rémi Carles CNRS & Univ. Montpellier Rémi Carles (Montpellier) Splitting for semiclassical NLS 1 / 21 Splitting for NLS i t u + 1 2 u = f ( u 2) u,
On time splitting for NLS in the semiclassical regime Rémi Carles CNRS & Univ. Montpellier Rémi Carles (Montpellier) Splitting for semiclassical NLS 1 / 21 Splitting for NLS i t u + 1 2 u = f ( u 2) u,
FYSMEK1110 Eksamensverksted 23. Mai :15-18:00 Oppgave 1 (maks. 45 minutt)
 FYSMEK1110 Eksamensverksted 23. Mai 2018 14:15-18:00 Oppgave 1 (maks. 45 minutt) Page 1 of 9 Svar, eksempler, diskusjon og gode råd fra studenter (30 min) Hva får dere poeng for? Gode råd fra forelesere
FYSMEK1110 Eksamensverksted 23. Mai 2018 14:15-18:00 Oppgave 1 (maks. 45 minutt) Page 1 of 9 Svar, eksempler, diskusjon og gode råd fra studenter (30 min) Hva får dere poeng for? Gode råd fra forelesere
32.2. Linear Multistep Methods. Introduction. Prerequisites. Learning Outcomes
 Linear Multistep Methods 32.2 Introduction In the previous Section we saw two methods (Euler and trapezium) for approximating the solutions of certain initial value problems. In this Section we will see
Linear Multistep Methods 32.2 Introduction In the previous Section we saw two methods (Euler and trapezium) for approximating the solutions of certain initial value problems. In this Section we will see
HØGSKOLEN I NARVIK - SIVILINGENIØRUTDANNINGEN
 HØGSKOLEN I NARVIK - SIVILINGENIØRUTDANNINGEN EKSAMEN I FAGET STE 6243 MODERNE MATERIALER KLASSE: 5ID DATO: 7 Oktober 2005 TID: 900-200, 3 timer ANTALL SIDER: 7 (inklusiv Appendix: tabell og formler) TILLATTE
HØGSKOLEN I NARVIK - SIVILINGENIØRUTDANNINGEN EKSAMEN I FAGET STE 6243 MODERNE MATERIALER KLASSE: 5ID DATO: 7 Oktober 2005 TID: 900-200, 3 timer ANTALL SIDER: 7 (inklusiv Appendix: tabell og formler) TILLATTE
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSIEE I OSLO ØKONOMISK INSIU Eksamen i: ECON320/420 Mathematics 2: Calculus and Linear Algebra Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag:. desember 207 Sensur kunngjøres:
UNIVERSIEE I OSLO ØKONOMISK INSIU Eksamen i: ECON320/420 Mathematics 2: Calculus and Linear Algebra Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag:. desember 207 Sensur kunngjøres:
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON320/420 Mathematics 2: Calculus and linear algebra Exam: ECON320/420 Mathematics 2: Calculus and linear algebra Eksamensdag: Tirsdag 30. mai 207
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON320/420 Mathematics 2: Calculus and linear algebra Exam: ECON320/420 Mathematics 2: Calculus and linear algebra Eksamensdag: Tirsdag 30. mai 207
Eksamensoppgave i TMA4320 Introduksjon til vitenskapelige beregninger
 Institutt for matematiske fag Eksamensoppgave i TMA432 Introduksjon til vitenskapelige beregninger Faglig kontakt under eksamen: Anton Evgrafov Tlf: 453 163 Eksamensdato: 8. august 217 Eksamenstid (fra
Institutt for matematiske fag Eksamensoppgave i TMA432 Introduksjon til vitenskapelige beregninger Faglig kontakt under eksamen: Anton Evgrafov Tlf: 453 163 Eksamensdato: 8. august 217 Eksamenstid (fra
Endelig ikke-røyker for Kvinner! (Norwegian Edition)
 Endelig ikke-røyker for Kvinner! (Norwegian Edition) Allen Carr Click here if your download doesn"t start automatically Endelig ikke-røyker for Kvinner! (Norwegian Edition) Allen Carr Endelig ikke-røyker
Endelig ikke-røyker for Kvinner! (Norwegian Edition) Allen Carr Click here if your download doesn"t start automatically Endelig ikke-røyker for Kvinner! (Norwegian Edition) Allen Carr Endelig ikke-røyker
TMA4329 Intro til vitensk. beregn. V2017
 Norges teknisk naturvitenskapelige universitet Institutt for Matematiske Fag TMA439 Intro til vitensk. beregn. V17 ving 4 [S]T. Sauer, Numerical Analysis, Second International Edition, Pearson, 14 Teorioppgaver
Norges teknisk naturvitenskapelige universitet Institutt for Matematiske Fag TMA439 Intro til vitensk. beregn. V17 ving 4 [S]T. Sauer, Numerical Analysis, Second International Edition, Pearson, 14 Teorioppgaver
0:7 0:2 0:1 0:3 0:5 0:2 0:1 0:4 0:5 P = 0:56 0:28 0:16 0:38 0:39 0:23
 UTKAST ENGLISH VERSION EKSAMEN I: MOT100A STOKASTISKE PROSESSER VARIGHET: 4 TIMER DATO: 16. februar 2006 TILLATTE HJELPEMIDLER: Kalkulator; Tabeller og formler i statistikk (Tapir forlag): Rottman: Matematisk
UTKAST ENGLISH VERSION EKSAMEN I: MOT100A STOKASTISKE PROSESSER VARIGHET: 4 TIMER DATO: 16. februar 2006 TILLATTE HJELPEMIDLER: Kalkulator; Tabeller og formler i statistikk (Tapir forlag): Rottman: Matematisk
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Utsatt eksamen i: ECON420 Matematikk 2: Matematisk analyse og lineær algebra Postponed exam: ECON420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Mandag
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Utsatt eksamen i: ECON420 Matematikk 2: Matematisk analyse og lineær algebra Postponed exam: ECON420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Mandag
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Utsatt eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Postponed exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag:
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Utsatt eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Postponed exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag:
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Mandag 8. desember
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON320/420 Matematikk 2: Matematisk analyse og lineær algebra Exam: ECON320/420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Mandag 8. desember
Emneevaluering GEOV272 V17
 Emneevaluering GEOV272 V17 Studentenes evaluering av kurset Svarprosent: 36 % (5 av 14 studenter) Hvilket semester er du på? Hva er ditt kjønn? Er du...? Er du...? - Annet PhD Candidate Samsvaret mellom
Emneevaluering GEOV272 V17 Studentenes evaluering av kurset Svarprosent: 36 % (5 av 14 studenter) Hvilket semester er du på? Hva er ditt kjønn? Er du...? Er du...? - Annet PhD Candidate Samsvaret mellom
Verifiable Secret-Sharing Schemes
 Aarhus University Verifiable Secret-Sharing Schemes Irene Giacomelli joint work with Ivan Damgård, Bernardo David and Jesper B. Nielsen Aalborg, 30th June 2014 Verifiable Secret-Sharing Schemes Aalborg,
Aarhus University Verifiable Secret-Sharing Schemes Irene Giacomelli joint work with Ivan Damgård, Bernardo David and Jesper B. Nielsen Aalborg, 30th June 2014 Verifiable Secret-Sharing Schemes Aalborg,
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 1 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT BOKMÅL Utsatt eksamen i: ECON2915 Vekst og næringsstruktur Eksamensdag: 07.12.2012 Tid for eksamen: kl. 09:00-12:00 Oppgavesettet er på 5 sider Tillatte hjelpemidler:
1 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT BOKMÅL Utsatt eksamen i: ECON2915 Vekst og næringsstruktur Eksamensdag: 07.12.2012 Tid for eksamen: kl. 09:00-12:00 Oppgavesettet er på 5 sider Tillatte hjelpemidler:
Han Ola of Han Per: A Norwegian-American Comic Strip/En Norsk-amerikansk tegneserie (Skrifter. Serie B, LXIX)
 Han Ola of Han Per: A Norwegian-American Comic Strip/En Norsk-amerikansk tegneserie (Skrifter. Serie B, LXIX) Peter J. Rosendahl Click here if your download doesn"t start automatically Han Ola of Han Per:
Han Ola of Han Per: A Norwegian-American Comic Strip/En Norsk-amerikansk tegneserie (Skrifter. Serie B, LXIX) Peter J. Rosendahl Click here if your download doesn"t start automatically Han Ola of Han Per:
Eksamen i TMA4190 Mangfoldigheter Onsdag 4 juni, Tid :
 Norges teknisk-naturvitenskapelige universitet Institutt for matematiske fag SOLUTIONS Eksamen i TMA4190 Mangfoldigheter Onsdag 4 juni, 2013. Tid : 09.00 13.00 Oppgave 1 a) La U R n være enhetsdisken x
Norges teknisk-naturvitenskapelige universitet Institutt for matematiske fag SOLUTIONS Eksamen i TMA4190 Mangfoldigheter Onsdag 4 juni, 2013. Tid : 09.00 13.00 Oppgave 1 a) La U R n være enhetsdisken x
Eksamen i FY3466 KVANTEFELTTEORI II Tirsdag 20. mai :00 13:00
 NTNU Side 1 av 3 Institutt for fysikk Faglig kontakt under eksamen: Professor Kåre Olaussen Telefon: 9 36 52 eller 45 43 71 70 Eksamen i FY3466 KVANTEFELTTEORI II Tirsdag 20. mai 2008 09:00 13:00 Tillatte
NTNU Side 1 av 3 Institutt for fysikk Faglig kontakt under eksamen: Professor Kåre Olaussen Telefon: 9 36 52 eller 45 43 71 70 Eksamen i FY3466 KVANTEFELTTEORI II Tirsdag 20. mai 2008 09:00 13:00 Tillatte
Oppgave 1a Definer følgende begreper: Nøkkel, supernøkkel og funksjonell avhengighet.
 TDT445 Øving 4 Oppgave a Definer følgende begreper: Nøkkel, supernøkkel og funksjonell avhengighet. Nøkkel: Supernøkkel: Funksjonell avhengighet: Data i en database som kan unikt identifisere (et sett
TDT445 Øving 4 Oppgave a Definer følgende begreper: Nøkkel, supernøkkel og funksjonell avhengighet. Nøkkel: Supernøkkel: Funksjonell avhengighet: Data i en database som kan unikt identifisere (et sett
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON1910 Poverty and distribution in developing countries Exam: ECON1910 Poverty and distribution in developing countries Eksamensdag: 1. juni 2011 Sensur
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON1910 Poverty and distribution in developing countries Exam: ECON1910 Poverty and distribution in developing countries Eksamensdag: 1. juni 2011 Sensur
Exercise 1: Phase Splitter DC Operation
 Exercise 1: DC Operation When you have completed this exercise, you will be able to measure dc operating voltages and currents by using a typical transistor phase splitter circuit. You will verify your
Exercise 1: DC Operation When you have completed this exercise, you will be able to measure dc operating voltages and currents by using a typical transistor phase splitter circuit. You will verify your
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON360/460 Samfunnsøkonomisk lønnsomhet og økonomisk politikk Exam: ECON360/460 - Resource allocation and economic policy Eksamensdag: Fredag 2. november
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON360/460 Samfunnsøkonomisk lønnsomhet og økonomisk politikk Exam: ECON360/460 - Resource allocation and economic policy Eksamensdag: Fredag 2. november
UNIVERSITETET I OSLO
 UNIVERSITETET I OSLO Det matematisk-naturvitenskapelige fakultet Eksamen i INF 3230 Formell modellering og analyse av kommuniserende systemer Eksamensdag: 4. april 2008 Tid for eksamen: 9.00 12.00 Oppgavesettet
UNIVERSITETET I OSLO Det matematisk-naturvitenskapelige fakultet Eksamen i INF 3230 Formell modellering og analyse av kommuniserende systemer Eksamensdag: 4. april 2008 Tid for eksamen: 9.00 12.00 Oppgavesettet
Exam in Quantum Mechanics (phys201), 2010, Allowed: Calculator, standard formula book and up to 5 pages of own handwritten notes.
 Exam in Quantum Mechanics (phys01), 010, There are 3 problems, 1 3. Each problem has several sub problems. The number of points for each subproblem is marked. Allowed: Calculator, standard formula book
Exam in Quantum Mechanics (phys01), 010, There are 3 problems, 1 3. Each problem has several sub problems. The number of points for each subproblem is marked. Allowed: Calculator, standard formula book
Lipschitz Metrics for Non-smooth evolutions
 Lipschitz Metrics for Non-smooth evolutions Alberto Bressan Department of Mathematics, Penn State University bressan@math.psu.edu Alberto Bressan (Penn State) Nonsmooth evolutions 1 / 36 Well-posedness
Lipschitz Metrics for Non-smooth evolutions Alberto Bressan Department of Mathematics, Penn State University bressan@math.psu.edu Alberto Bressan (Penn State) Nonsmooth evolutions 1 / 36 Well-posedness
Hvor mye praktisk kunnskap har du tilegnet deg på dette emnet? (1 = ingen, 5 = mye)
 INF247 Er du? Er du? - Annet Ph.D. Student Hvor mye teoretisk kunnskap har du tilegnet deg på dette emnet? (1 = ingen, 5 = mye) Hvor mye praktisk kunnskap har du tilegnet deg på dette emnet? (1 = ingen,
INF247 Er du? Er du? - Annet Ph.D. Student Hvor mye teoretisk kunnskap har du tilegnet deg på dette emnet? (1 = ingen, 5 = mye) Hvor mye praktisk kunnskap har du tilegnet deg på dette emnet? (1 = ingen,
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Utsatt eksamen i: ECON420 Matematikk 2: Matematisk analyse og lineær algebra Postponed exam: ECON420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Mandag
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Utsatt eksamen i: ECON420 Matematikk 2: Matematisk analyse og lineær algebra Postponed exam: ECON420 Mathematics 2: Calculus and Linear Algebra Eksamensdag: Mandag
STILLAS - STANDARD FORSLAG FRA SEF TIL NY STILLAS - STANDARD
 FORSLAG FRA SEF TIL NY STILLAS - STANDARD 1 Bakgrunnen for dette initiativet fra SEF, er ønsket om å gjøre arbeid i høyden tryggere / sikrere. Både for stillasmontører og brukere av stillaser. 2 Reviderte
FORSLAG FRA SEF TIL NY STILLAS - STANDARD 1 Bakgrunnen for dette initiativet fra SEF, er ønsket om å gjøre arbeid i høyden tryggere / sikrere. Både for stillasmontører og brukere av stillaser. 2 Reviderte
Den som gjør godt, er av Gud (Multilingual Edition)
 Den som gjør godt, er av Gud (Multilingual Edition) Arne Jordly Click here if your download doesn"t start automatically Den som gjør godt, er av Gud (Multilingual Edition) Arne Jordly Den som gjør godt,
Den som gjør godt, er av Gud (Multilingual Edition) Arne Jordly Click here if your download doesn"t start automatically Den som gjør godt, er av Gud (Multilingual Edition) Arne Jordly Den som gjør godt,
Andrew Gendreau, Olga Rosenbaum, Anthony Taylor, Kenneth Wong, Karl Dusen
 Andrew Gendreau, Olga Rosenbaum, Anthony Taylor, Kenneth Wong, Karl Dusen The Process Goal Definition Data Collection Data Preprocessing EDA Choice of Variables Choice of Method(s) Performance Evaluation
Andrew Gendreau, Olga Rosenbaum, Anthony Taylor, Kenneth Wong, Karl Dusen The Process Goal Definition Data Collection Data Preprocessing EDA Choice of Variables Choice of Method(s) Performance Evaluation
May legally defined terms be useful for improved interoperability in the public sector?
 May legally defined terms be useful for improved interoperability in the public sector? Prof. Dag Wiese Schartum, Norwegian Research Center for Computers and Law, University of Oslo How may terms be legally
May legally defined terms be useful for improved interoperability in the public sector? Prof. Dag Wiese Schartum, Norwegian Research Center for Computers and Law, University of Oslo How may terms be legally
Oppgave 1. Norges teknisk-naturvitenskapelige universitet NTNU Institutt for fysikk EKSAMEN I: MNFFY 245 INNFØRING I KVANTEMEKANIKK
 Norges teknisk-naturvitenskapelige universitet NTNU Institutt for fysikk EKSAMEN I: MNFFY 45 INNFØRING I KVANTEMEKANIKK DATO: Fredag 4 desember TID: 9 5 Antall vekttall: 4 Antall sider: 5 Tillatte hjelpemidler:
Norges teknisk-naturvitenskapelige universitet NTNU Institutt for fysikk EKSAMEN I: MNFFY 45 INNFØRING I KVANTEMEKANIKK DATO: Fredag 4 desember TID: 9 5 Antall vekttall: 4 Antall sider: 5 Tillatte hjelpemidler:
Continuity. Subtopics
 0 Cotiuity Chapter 0: Cotiuity Subtopics.0 Itroductio (Revisio). Cotiuity of a Fuctio at a Poit. Discotiuity of a Fuctio. Types of Discotiuity.4 Algebra of Cotiuous Fuctios.5 Cotiuity i a Iterval.6 Cotiuity
0 Cotiuity Chapter 0: Cotiuity Subtopics.0 Itroductio (Revisio). Cotiuity of a Fuctio at a Poit. Discotiuity of a Fuctio. Types of Discotiuity.4 Algebra of Cotiuous Fuctios.5 Cotiuity i a Iterval.6 Cotiuity
Energy Dissipation in Hybrid Stars. Sophia Han. Washington University
 Energy Dissipation in Hybrid Stars Sophia Han Washington University Texas Symposium December 8-13, 2013 Mark Alford, Sophia Han, Kai Schwenzer 1 Context Nuclear Matter Crust Quark Matter Core -Sharp interface:
Energy Dissipation in Hybrid Stars Sophia Han Washington University Texas Symposium December 8-13, 2013 Mark Alford, Sophia Han, Kai Schwenzer 1 Context Nuclear Matter Crust Quark Matter Core -Sharp interface:
EKSAMEN I FAG TTT4110 Informasjons- og signalteori. Norsk tekst på oddetalls-sider. (English text on even numbered pages.)
 Side/Page 1 av/of 8 + 3 sider vedlegg + enclosure, 3 pages NORGES TEKNISK-NATURVITENSKAPELIGE UNIVERSITET INSTITUTT FOR ELEKTRONIKK OG TELEKOMMUNIKASJON Signalbehandling Faglig kontakt under eksamen: Navn:
Side/Page 1 av/of 8 + 3 sider vedlegg + enclosure, 3 pages NORGES TEKNISK-NATURVITENSKAPELIGE UNIVERSITET INSTITUTT FOR ELEKTRONIKK OG TELEKOMMUNIKASJON Signalbehandling Faglig kontakt under eksamen: Navn:
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON3120/4120 Mathematics 2: Calculus an linear algebra Exam: ECON3120/4120 Mathematics 2: Calculus an linear algebra Eksamensag: Tirsag 3. juni 2008
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON3120/4120 Mathematics 2: Calculus an linear algebra Exam: ECON3120/4120 Mathematics 2: Calculus an linear algebra Eksamensag: Tirsag 3. juni 2008
HONSEL process monitoring
 6 DMSD has stood for process monitoring in fastening technology for more than 25 years. HONSEL re- rivet processing back in 990. DMSD 2G has been continuously improved and optimised since this time. All
6 DMSD has stood for process monitoring in fastening technology for more than 25 years. HONSEL re- rivet processing back in 990. DMSD 2G has been continuously improved and optimised since this time. All
Information search for the research protocol in IIC/IID
 Information search for the research protocol in IIC/IID 1 Medical Library, 2013 Library services for students working with the research protocol and thesis (hovedoppgaven) Open library courses: http://www.ntnu.no/ub/fagside/medisin/medbiblkurs
Information search for the research protocol in IIC/IID 1 Medical Library, 2013 Library services for students working with the research protocol and thesis (hovedoppgaven) Open library courses: http://www.ntnu.no/ub/fagside/medisin/medbiblkurs
Start MATLAB. Start NUnet Applications Statistical and Computational packages MATLAB Release 13 MATLAB 6.5
 Start MATLAB Start NUnet Applications Statistical and Computational packages MATLAB Release 13 MATLAB 6.5 Prompt >> will appear in the command window Today: MATLAB overview In-class HW: Chapter 1, Problems
Start MATLAB Start NUnet Applications Statistical and Computational packages MATLAB Release 13 MATLAB 6.5 Prompt >> will appear in the command window Today: MATLAB overview In-class HW: Chapter 1, Problems
GEF2200 Atmosfærefysikk 2017
 GEF2200 Atmosfærefysikk 2017 Løsningsforslag til sett 3 Oppgaver hentet fra boka Wallace and Hobbs (2006) er merket WH06 WH06 3.18r Unsaturated air is lifted (adiabatically): The rst pair of quantities
GEF2200 Atmosfærefysikk 2017 Løsningsforslag til sett 3 Oppgaver hentet fra boka Wallace and Hobbs (2006) er merket WH06 WH06 3.18r Unsaturated air is lifted (adiabatically): The rst pair of quantities
UNIVERSITY OF OSLO DEPARTMENT OF ECONOMICS
 UNIVERSITY OF OSLO DEPARTMENT OF ECONOMICS English Exam: ECON2915 Economic Growth Date of exam: 25.11.2014 Grades will be given: 16.12.2014 Time for exam: 09.00 12.00 The problem set covers 3 pages Resources
UNIVERSITY OF OSLO DEPARTMENT OF ECONOMICS English Exam: ECON2915 Economic Growth Date of exam: 25.11.2014 Grades will be given: 16.12.2014 Time for exam: 09.00 12.00 The problem set covers 3 pages Resources
Level-Rebuilt B-Trees
 Gerth Stølting Brodal BRICS University of Aarhus Pankaj K. Agarwal Lars Arge Jeffrey S. Vitter Center for Geometric Computing Duke University August 1998 1 B-Trees Bayer, McCreight 1972 Level 2 Level 1
Gerth Stølting Brodal BRICS University of Aarhus Pankaj K. Agarwal Lars Arge Jeffrey S. Vitter Center for Geometric Computing Duke University August 1998 1 B-Trees Bayer, McCreight 1972 Level 2 Level 1
Gir vi de resterende 2 oppgavene til én prosess vil alle sitte å vente på de to potensielt tidskrevende prosessene.
 Figure over viser 5 arbeidsoppgaver som hver tar 0 miutter å utføre av e arbeider. (E oppgave ka ku utføres av é arbeider.) Hver pil i figure betyr at oppgave som blir pekt på ikke ka starte før oppgave
Figure over viser 5 arbeidsoppgaver som hver tar 0 miutter å utføre av e arbeider. (E oppgave ka ku utføres av é arbeider.) Hver pil i figure betyr at oppgave som blir pekt på ikke ka starte før oppgave
melting ECMI Modelling week 2008 Modelling and simulation of ice/snow melting Sabrina Wandl - University of Linz Tuomo Mäki-Marttunen - Tampere UT
 and and ECMI week 2008 Outline and Problem Description find model for processes consideration of effects caused by presence of salt point and numerical solution and and heat equations liquid phase: T L
and and ECMI week 2008 Outline and Problem Description find model for processes consideration of effects caused by presence of salt point and numerical solution and and heat equations liquid phase: T L
NO X -chemistry modeling for coal/biomass CFD
 NO X -chemistry modeling for coal/biomass CFD Jesper Møller Pedersen 1, Larry Baxter 2, Søren Knudsen Kær 3, Peter Glarborg 4, Søren Lovmand Hvid 1 1 DONG Energy, Denmark 2 BYU, USA 3 AAU, Denmark 4 DTU,
NO X -chemistry modeling for coal/biomass CFD Jesper Møller Pedersen 1, Larry Baxter 2, Søren Knudsen Kær 3, Peter Glarborg 4, Søren Lovmand Hvid 1 1 DONG Energy, Denmark 2 BYU, USA 3 AAU, Denmark 4 DTU,
Dagens tema: Eksempel Klisjéer (mønstre) Tommelfingerregler
 UNIVERSITETET I OSLO INF1300 Introduksjon til databaser Dagens tema: Eksempel Klisjéer (mønstre) Tommelfingerregler Institutt for informatikk Dumitru Roman 1 Eksempel (1) 1. The system shall give an overview
UNIVERSITETET I OSLO INF1300 Introduksjon til databaser Dagens tema: Eksempel Klisjéer (mønstre) Tommelfingerregler Institutt for informatikk Dumitru Roman 1 Eksempel (1) 1. The system shall give an overview
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT
 UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON2915 Vekst og næringsstruktur Exam: ECON2915 - Growth and business structure Eksamensdag: Fredag 2. desember 2005 Sensur kunngjøres: 20. desember
UNIVERSITETET I OSLO ØKONOMISK INSTITUTT Eksamen i: ECON2915 Vekst og næringsstruktur Exam: ECON2915 - Growth and business structure Eksamensdag: Fredag 2. desember 2005 Sensur kunngjøres: 20. desember
PATIENCE TÅLMODIGHET. Is the ability to wait for something. Det trenger vi når vi må vente på noe
 CARING OMSORG Is when we show that we care about others by our actions or our words Det er når vi viser at vi bryr oss om andre med det vi sier eller gjør PATIENCE TÅLMODIGHET Is the ability to wait for
CARING OMSORG Is when we show that we care about others by our actions or our words Det er når vi viser at vi bryr oss om andre med det vi sier eller gjør PATIENCE TÅLMODIGHET Is the ability to wait for
On Capacity Planning for Minimum Vulnerability
 On Capacity Planning for Minimum Vulnerability Alireza Bigdeli Ali Tizghadam Alberto Leon-Garcia University of Toronto DRCN - October 2011 Kakow - Poland 1 Outline Introduction Network Criticality and
On Capacity Planning for Minimum Vulnerability Alireza Bigdeli Ali Tizghadam Alberto Leon-Garcia University of Toronto DRCN - October 2011 Kakow - Poland 1 Outline Introduction Network Criticality and
ECON3120/4120 Mathematics 2, spring 2004 Problem solutions for the seminar on 5 May Old exam problems
 Department of Economics May 004 Arne Strøm ECON0/40 Mathematics, spring 004 Problem solutions for the seminar on 5 May 004 (For practical reasons (read laziness, most of the solutions this time are in
Department of Economics May 004 Arne Strøm ECON0/40 Mathematics, spring 004 Problem solutions for the seminar on 5 May 004 (For practical reasons (read laziness, most of the solutions this time are in
EKSAMENSOPPGAVE I BI2034 Samfunnsøkologi EXAMINATION IN: BI Community ecology
 Norges teknisk-naturvitenskapelige universitet Institutt for Biologi EKSAMENSOPPGAVE I BI2034 Samfunnsøkologi EXAMINATION IN: BI2034 - Community ecology - Faglig kontakt under eksamen/contact person/subject
Norges teknisk-naturvitenskapelige universitet Institutt for Biologi EKSAMENSOPPGAVE I BI2034 Samfunnsøkologi EXAMINATION IN: BI2034 - Community ecology - Faglig kontakt under eksamen/contact person/subject
Hvordan føre reiseregninger i Unit4 Business World Forfatter:
 Hvordan føre reiseregninger i Unit4 Business World Forfatter: dag.syversen@unit4.com Denne e-guiden beskriver hvordan du registrerer en reiseregning med ulike typer utlegg. 1. Introduksjon 2. Åpne vinduet
Hvordan føre reiseregninger i Unit4 Business World Forfatter: dag.syversen@unit4.com Denne e-guiden beskriver hvordan du registrerer en reiseregning med ulike typer utlegg. 1. Introduksjon 2. Åpne vinduet
EKSAMENSOPPGAVE I FAG TKP 4105
 EKSAMENSOPPGAVE I FAG TKP 4105 Faglig kontakt under eksamen: Sigurd Skogestad Tlf: 913 71669 (May-Britt Hägg Tlf: 930 80834) Eksamensdato: 08.12.11 Eksamenstid: 09:00 13:00 7,5 studiepoeng Tillatte hjelpemidler:
EKSAMENSOPPGAVE I FAG TKP 4105 Faglig kontakt under eksamen: Sigurd Skogestad Tlf: 913 71669 (May-Britt Hägg Tlf: 930 80834) Eksamensdato: 08.12.11 Eksamenstid: 09:00 13:00 7,5 studiepoeng Tillatte hjelpemidler:
Oppgave. føden)? i tråd med
 Oppgaver Sigurd Skogestad, Eksamen septek 16. des. 2013 Oppgave 2. Destillasjon En destillasjonskolonne har 7 teoretiske trinn (koker + 3 ideelle plater under føden + 2 ideellee plater over føden + partielll
Oppgaver Sigurd Skogestad, Eksamen septek 16. des. 2013 Oppgave 2. Destillasjon En destillasjonskolonne har 7 teoretiske trinn (koker + 3 ideelle plater under føden + 2 ideellee plater over føden + partielll
An oscillatory bifurcation from infinity, and
 Nonlinear differ. equ. appl. 15 (28), 335 345 121 9722/8/3335 11, DOI 1.17/s3-8-724-1 c 28 Birkhäuser Verlag Basel/Switzerland An oscillatory bifurcation from infinity, and from zero Philip Korman Abstract.
Nonlinear differ. equ. appl. 15 (28), 335 345 121 9722/8/3335 11, DOI 1.17/s3-8-724-1 c 28 Birkhäuser Verlag Basel/Switzerland An oscillatory bifurcation from infinity, and from zero Philip Korman Abstract.
FIRST LEGO League. Härnösand 2012
 FIRST LEGO League Härnösand 2012 Presentasjon av laget IES Dragons Vi kommer fra Härnosänd Snittalderen på våre deltakere er 11 år Laget består av 4 jenter og 4 gutter. Vi representerer IES i Sundsvall
FIRST LEGO League Härnösand 2012 Presentasjon av laget IES Dragons Vi kommer fra Härnosänd Snittalderen på våre deltakere er 11 år Laget består av 4 jenter og 4 gutter. Vi representerer IES i Sundsvall
Minimumskrav bør være å etablere at samtykke ikke bare må være gitt frivillig, men også informert.
 Sensorveiledning SPED1200 vår 2018 NB! Studentene har fått beskjed om at de kan bruke engelske ord og uttrykk i besvarelsen uten at dette betraktes som negativt. Dette skal altså ikke trekke ned i vurderingen.
Sensorveiledning SPED1200 vår 2018 NB! Studentene har fått beskjed om at de kan bruke engelske ord og uttrykk i besvarelsen uten at dette betraktes som negativt. Dette skal altså ikke trekke ned i vurderingen.
Periodic solutions for a class of non-autonomous differential delay equations
 Nonlinear Differ. Equ. Appl. 16 (2009), 793 809 c 2009 Birkhäuser Verlag Basel/Switzerland 1021-9722/09/060793-17 published online July 4, 2009 DOI 10.1007/s00030-009-0035-8 Nonlinear Differential Equations
Nonlinear Differ. Equ. Appl. 16 (2009), 793 809 c 2009 Birkhäuser Verlag Basel/Switzerland 1021-9722/09/060793-17 published online July 4, 2009 DOI 10.1007/s00030-009-0035-8 Nonlinear Differential Equations
Finite Elements Methods. Formulary for Prof. Estor's exam
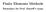 Finite Elements Methods Formulary for Prof. Estor's exam Finite Element Method in General One wants to obtain the equilibrium eqautions for the body, discretized by nite elements in the form M Ü + C U
Finite Elements Methods Formulary for Prof. Estor's exam Finite Element Method in General One wants to obtain the equilibrium eqautions for the body, discretized by nite elements in the form M Ü + C U
